Thermodynamic Properties
Within thermodynamics, a physical property is any property that is measurable, and whose value describes a state of a physical system. Our goal here will be to introduce thermodynamic properties, that are used in engineering thermodynamics. These properties will be further applied to energy systems and finally to thermal or nuclear power plants.
In general, thermodynamic properties can be divided into two general classes:
- Extensive properties: An extensive property is dependent upon the amount of mass present or upon the size or extent of a system. For example, the following properties are extensive:
- Intensive property: An intensive property is independent of the amount of mass and may vary from place to place within the system at any moment. For example, the following properties are extensive:
- Compressibility
- Density
- Specific Enthalpy
- Specific Entropy
- Specific Heat Capacity
- Pressure
- Temperature
- Thermal Conductivity
- Thermal Expansion
- Vapor Quality
- Specific Volume
Specific Properties
Specific properties of material are derived from other intensive and extensive properties of that material. For example, the density of water is an intensive property and can be derived from measurements of the mass of a water volume (an extensive property) divided by the volume (another extensive property). Also heat capacity, which is an extensive property of a system can be derived from heat capacity, Cp, and the mass of the system. Dividing these extensive properties gives the specific heat capacity, cp, which is an intensive property.
Specific properties are often used in reference tables as a means of recording material data in a manner that is independent of size or mass. They are very useful for making comparisons about one attribute while cancelling out the effect of variations in another attribute.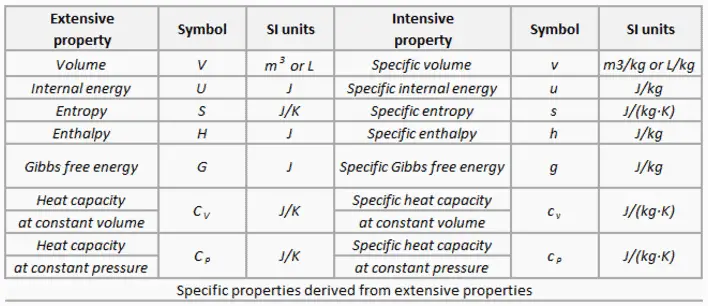
Mass vs. Weight
One of the most familiar forces is the weight of a body, which is the gravitational force that the earth exerts on the body. In general, gravitation is a natural phenomenon by which all things with mass are brought toward one another. The terms mass and weight are often confused with one another, but it is important to distinguish between them. It is absolutely essential to understand clearly the distinctions between these two physical quantities.
The mass of a certain body will remain constant even if the gravitational acceleration acting upon that body changes. For example, on earth an object has a certain mass and a certain weight. When the same object is placed in outer space, away from the earth’s gravitational field, its mass remains the same, but it is now in a “weightless” condition. This means in this condition it will weight zero, because gravitational acceleration and, thus, force will equal to zero.
Mass and weight are related: Bodies having large mass also have large weight. A large stone is hard to throw because of its large mass, and hard to lift off the ground because of its large weight. To understand the relationship between mass and weight, consider a freely falling stone, that has an acceleration of magnitude g (g = 9.81 m/s2 is the acceleration due to Earth’s gravitational field). Newton’s second law tells us that a force must act to produce this acceleration. If a 1 kilogram stone falls with an acceleration of the required force has magnitude:
F = ma = 1 [kg] x 9.81 [m/s2] = 9.8 [kg m/s2] = 9.8 N
The force that makes the body accelerate downward is its weight. Any body near the surface of the earth that has a mass of 1 kg must have a weight of 9.8 N to give it the acceleration we observe when it is in free fall.
Example: The weight of a stone on the Earth, on the Mars and on the Moon
Weight of a stone on the Earth
The acceleration due to Earth’s gravitational field is gEarth = 9.81 m/s2.The weight of a stone with mass 1 kg on the Earth can be calculated as:
FEarth = 1 [kg] x 9.81 [m/s2] = 9.8 [kg m/s2] = 9.8 N
Weight of a stone on the Mars
The acceleration of gravity on the Mars is approximately 38% of the acceleration of gravity on the earth. The acceleration due to Moon’s gravitational field is gMars = 3.71 m/s2.
Therefore the weight of the same stone with mass 1 kg on the Mars is:
FMoon = 1 [kg] x 3.71 [m/s2] = 3.71 [kg m/s2] = 3.71 N
Weight of a stone on the Moon
The acceleration of gravity on the Moon is approximately 1/6 of the acceleration of gravity on the earth. The acceleration due to Moon’s gravitational field is gMoon = 1.62 m/s2.
Therefore the weight of the same stone with mass 1 kg on the Moon is:
FMoon = 1 [kg] x 1.62 [m/s2] = 1.62 [kg m/s2] = 1.62 N
The Standard Kilogram
The usual symbol for mass is m and its SI unit is the kilogram. The SI standard of mass is a cylinder of platinum and iridium that is kept at the International Bureau of Weights and Measures near Paris and assigned, by international agreement, a mass of 1 kilogram.
Toggle: Relativistic Mass
While the mass is normally considered to be an unchanging property of an object, at speeds approaching the speed of light one must consider the increase in the relativistic mass. The relativistic definition of momentum is sometimes interpreted as an increase in the mass of an object. In this interpretation, a particle can have a relativistic mass, mrel. The increase in effective mass with speed is given by the expression:
In this “mass-increase” formula, m is referred to as the rest mass of the object. It follows from this formula, that an object with nonzero rest mass cannot travel at the speed of light. As the object approaches the speed of light, the object’s momentum increase without bound. On the other hand, when the relative velocity is zero, the Lorentz factor is simply equal to 1, and the relativistic mass is reduced to the rest mass. With this interpretation, the mass of an object appears to increase as its speed increases. In must be added, many physicists believe an object has only one mass (its rest mass), and that it is only the momentum that increases with speed.
The mass of an object is a fundamental property of the object. It is a numerical measure of its inertia and the measure of an object’s resistance to acceleration when a force is applied. It is also a fundamental measure of the amount of matter in the object. The greater the mass, the greater the force needed to cause a given acceleration. This is reflected in Newton’s second law (F=ma).
The mass of a certain body will remain constant even if the gravitational acceleration acting upon that body changes. For example, on earth an object has a certain mass and a certain weight. When the same object is placed in outer space, away from the earth’s gravitational field, its mass remains the same, but it is now in a “weightless” condition. This means in this condition it will weight zero, because gravitational acceleration and, thus, force will equal to zero.
Mass and weight are related:
Bodies having large mass also have large weight. A large stone is hard to throw because of its large mass, and hard to lift off the ground because of its large weight. To understand the relationship between mass and weight, consider a freely falling stone, that has an acceleration of magnitude g (g = 9.81 m/s2 is the acceleration due to Earth’s gravitational field). Newton’s second law tells us that a force must act to produce this acceleration. If a 1 kilogram stone falls with an acceleration of the required force has magnitude:
F = ma = 1 [kg] x 9.81 [m/s2] = 9.8 [kg m/s2] = 9.8 N
The force that makes the body accelerate downward is its weight. Any body near the surface of the earth that has a mass of 1 kg must have a weight of 9.8 N to give it the acceleration we observe when it is in free fall.
Example: The weight of a stone on the Earth, on the Mars and on the Moon
Weight of a stone on the Earth
The acceleration due to Earth’s gravitational field is gEarth = 9.81 m/s2.The weight of a stone with mass 1 kg on the Earth can be calculated as:
FEarth = 1 [kg] x 9.81 [m/s2] = 9.8 [kg m/s2] = 9.8 N
Weight of a stone on the Mars
The acceleration of gravity on the Mars is approximately 38% of the acceleration of gravity on the earth. The acceleration due to Moon’s gravitational field is gMars = 3.71 m/s2.
Therefore the weight of the same stone with mass 1 kg on the Mars is:
FMoon = 1 [kg] x 3.71 [m/s2] = 3.71 [kg m/s2] = 3.71 N
Weight of a stone on the Moon
The acceleration of gravity on the Moon is approximately 1/6 of the acceleration of gravity on the earth. The acceleration due to Moon’s gravitational field is gMoon = 1.62 m/s2.
Therefore the weight of the same stone with mass 1 kg on the Moon is:
FMoon = 1 [kg] x 1.62 [m/s2] = 1.62 [kg m/s2] = 1.62 N
Principle of Equivalence:
An observer cannot determine, in any way whatsoever, whether the laboratory he occupies is in a uniform gravitational field or is in a reference frame that is accelerating relative to an inertial frame.
This led to an ambiguity as to what exactly is meant by the force of gravity and weight. A scale in an accelerating elevator cannot be distinguished from a scale in a gravitational field. Gravitational force and weight thereby became essentially frame-dependent quantities. According to the general theory of relativity, gravitational and inertial mass are not different properties of matter but two aspects of a fundamental and single property of matter.
In situations in which gravitation is absent but the chosen coordinate system is not inertial, but is accelerated with the observer, then g-forces and corresponding proper accelerations felt by observers in these coordinate systems are caused by the mechanical forces which resist their weight in such systems. The most realistic method of producing artificial gravity, for example aboard a space station, can be imitated in a rotating spaceship. Objects inside would be pushed toward the hull and they will have some weight. This weight is produced by fictitious forces or “inertial forces” which appear in all such accelerated coordinate systems. Unlike real gravity, which pulls towards a center of the planet, the centripetal force pushes towards the axis of rotation.
What is Volume
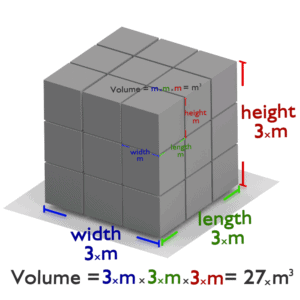Volume is a basic physical quantity. Volume is a derived quantity and it expresses the three dimensional extent of an object. Volume is often quantified numerically using the SI derived unit, the cubic metre. For example, the volume inside a sphere (that is the volume of a ball) is derived to be V = 4/3πr3, where r is the radius of the sphere. As another example, volume of a cube is equal to side times side times side. Since each side of a square is the same, it can simply be the length of one side cubed.
If a square has one side of 3 metres, the volume would be 3 metres times 3 metres times 3 metres, or 27 cubic metres.
The atom consist of a small but massive nucleus surrounded by a cloud of rapidly moving electrons. The nucleus is composed of protons and neutrons. Typical nuclear radii are of the order 10−14 m. Assuming spherical shape, nuclear radii can be calculated according to following formula:
r = r0 . A1/3
where r0 = 1.2 x 10-15 m = 1.2 fm
If we use this approximation, we therefore expect the volume of the nucleus to be of the order of 4/3πr3 or 7,23 ×10−45 m3 for hydrogen nuclei or 1721×10−45 m3 for 238U nuclei. These are volumes of nuclei and atomic nuclei (protons and neutrons) contains of about 99.95% of mass of atom.
See also: Is an atom an empty space?
What is Density
Density is defined as the mass per unit volume. It is an intensive property, which is mathematically defined as mass divided by volume:
ρ = m/V
In words, the density (ρ) of a substance is the total mass (m) of that substance divided by the total volume (V) occupied by that substance. The standard SI unit is kilograms per cubic meter (kg/m3). The Standard English unit is pounds mass per cubic foot (lbm/ft3). The density (ρ) of a substance is the reciprocal of its specific volume (ν).
ρ = m/V = 1/ρ
Specific volume is an intensive variable, whereas volume is an extensive variable. The standard unit for specific volume in the SI system is cubic meters per kilogram (m3/kg). The standard unit in the English system is cubic feet per pound mass (ft3/lbm).
The effect of temperature on the densities of liquids and solids is also very important. Most substances expand when heated and contract when cooled. However, the amount of expansion or contraction varies, depending on the material. This phenomenon is known as thermal expansion. The change in volume of a material which undergoes a temperature change is given by following relation:
where ∆T is the change in temperature, V is the original volume, ∆V is the change in volume, and αV is the coefficient of volume expansion.
It must be noted, there are exceptions from this rule. For example, water differs from most liquids in that it becomes less dense as it freezes. It has a maximum of density at 3.98 °C (1000 kg/m3), whereas the density of ice is 917 kg/m3. It differs by about 9% and therefore ice floats on liquid water
ρ = m/V = 1/
The specific volume () of a substance is the total volume (V) of that substance divided by the total mass (m) of that substance (volume per unit mass). It has units of cubic meter per kilogram (m3/kg).
Water and steam are a common medium because their properties are very well known. Their properties are tabulated in so called “Steam Tables”. In these tables the basic and key properties, such as pressure, temperature, enthalpy, density and specific heat, are tabulated along the vapor-liquid saturation curve as a function of both temperature and pressure.
The density (⍴) of any substance is the reciprocal of its specific volume ().
ρ = m/V = 1/
The specific volume () of a substance is the total volume (V) of that substance divided by the total mass (m) of that substance (volume per unit mass). It has units of cubic meter per kilogram (m3/kg).
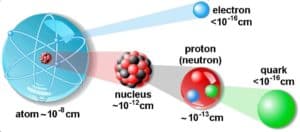
Density of Nuclear Matter
Nuclear density is the density of the nucleus of an atom. It is the ratio of mass per unit volume inside the nucleus. Since atomic nucleus carries most of atom’s mass and atomic nucleus is very small in comparison to entire atom, the nuclear density is very high.
The nuclear density for a typical nucleus can be approximately calculated from the size of the nucleus and from its mass. Typical nuclear radii are of the order 10−14 m. Assuming spherical shape, nuclear radii can be calculated according to following formula:
r = r0 . A1/3
where r0 = 1.2 x 10-15 m = 1.2 fm
For example, natural uranium consists primarily of isotope 238U (99.28%), therefore the atomic mass of uranium element is close to the atomic mass of 238U isotope (238.03u). Its radius of this nucleus will be:
r = r0 . A1/3 = 7.44 fm.
Assuming it is spherical, its volume will be:
V = 4πr3/3 = 1.73 x 10-42 m3.
The usual definition of nuclear density gives for its density:
ρnucleus = m / V = 238 x 1.66 x 10-27 / (1.73 x 10-42) = 2.3 x 1017 kg/m3.
Thus, the density of nuclear material is more than 2.1014 times greater than that of water. It is an immense density. The descriptive term nuclear density is also applied to situations where similarly high densities occur, such as within neutron stars. Such immense densities are also found in neutron stars.
What is Pressure
Pressure is a measure of the force exerted per unit area on the boundaries of a substance. The standard unit for pressure in the SI system is the Newton per square meter or pascal (Pa). Mathematically:
p = F/A
where
- p is the pressure
- F is the normal force
- A is the area of the boundary
Pascal is defined as force of 1N that is exerted on unit area.
- 1 Pascal = 1 N/m2
- 1 MPa 106 N/m2
- 1 bar 105 N/m2
- 1 kPa 103 N/m2
In general, pressure or the force exerted per unit area on the boundaries of a substance is caused by the collisions of the molecules of the substance with the boundaries of the system. As molecules hit the walls, they exert forces that try to push the walls outward. The forces resulting from all of these collisions cause the pressure exerted by a system on its surroundings. Pressure as an intensive variable is constant in a closed system. It really is only relevant in liquid or gaseous systems.
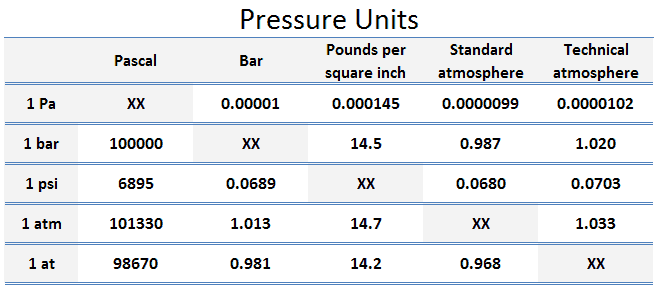
[xyz-ihs snippet=”pressure”]Pressure Scales – Pressure Units
Pascal – Unit of Pressure
As was discussed, the SI unit of pressure and stress is the pascal.
- 1 pascal 1 N/m2 = 1 kg / (m.s2)
Pascal is defined as one newton per square metre. However, for most engineering problems it is fairly small unit, so it is convenient to work with multiples of the pascal: the kPa, the bar, and the MPa.
- 1 MPa 106 N/m2
- 1 bar 105 N/m2
- 1 kPa 103 N/m2
The unit of measurement called standard atmosphere (atm) is defined as:
- 1 atm = 101.33 kPa
The standard atmosphere approximates to the average pressure at sea-level at the latitude 45° N. Note that, there is a difference between the standard atmosphere (atm) and the technical atmosphere (at).
A technical atmosphere is a non-SI unit of pressure equal to one kilogram-force per square centimeter.
- 1 at = 98.67 kPa
See also: Pound per square inch – psi
See also: Bar – Unit of Pressure
See also: Typical Pressures in Engineering
Absolute vs. Gauge Pressure
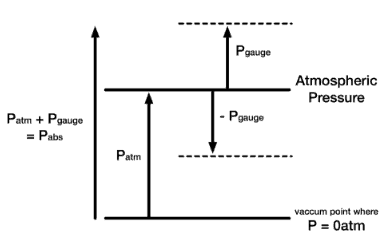
- Absolute Pressure. When pressure is measured relative to a perfect vacuum, it is called absolute pressure (psia). Pounds per square inch absolute (psia) is used to make it clear that the pressure is relative to a vacuum rather than the ambient atmospheric pressure. Since atmospheric pressure at sea level is around 101.3 kPa (14.7 psi), this will be added to any pressure reading made in air at sea level.
- Gauge Pressure. When pressure is measured relative to atmospheric pressure (14.7 psi), it is called gauge pressure (psig). The term gauge pressure is applied when the pressure in the system is greater than the local atmospheric pressure, patm. The latter pressure scale was developed because almost all pressure gauges register zero when open to the atmosphere. Gauge pressures are positive if they are above atmospheric pressure and negative if they are below atmospheric pressure.
pgauge = pabsolute – pabsolute; atm
- Atmospheric Pressure. Atmospheric pressure is the pressure in the surrounding air at – or “close” to – the surface of the earth. The atmospheric pressure varies with temperature and altitude above sea level. The Standard Atmospheric Pressure approximates to the average pressure at sea-level at the latitude 45° N. The Standard Atmospheric Pressure is defined at sea-level at 273oK (0oC) and is:
- 101325 Pa
- 1.01325 bar
- 14.696 psi
- 760 mmHg
- 760 torr
- Negative Gauge Pressure – Vacuum Pressure. When the local atmospheric pressure is greater than the pressure in the system, the term vacuum pressure is used. A perfect vacuum would correspond to absolute zero pressure. It is certainly possible to have a negative gauge pressure, but not possible to have a negative absolute pressure. For instance, an absolute pressure of 80 kPa may be described as a gauge pressure of −21 kPa (i.e., 21 kPa below an atmospheric pressure of 101 kPa).
pvacuum = pabsolute; atm – pabsolute
For example, a car tire pumped up to 2.5 atm (36.75 psig) above local atmospheric pressure (let say 1 atm or 14.7 psia locally), will have an absolute pressure of 2.5 + 1 = 3.5 atm (36.75 + 14.7 = 51.45 psia or 36.75 psig).
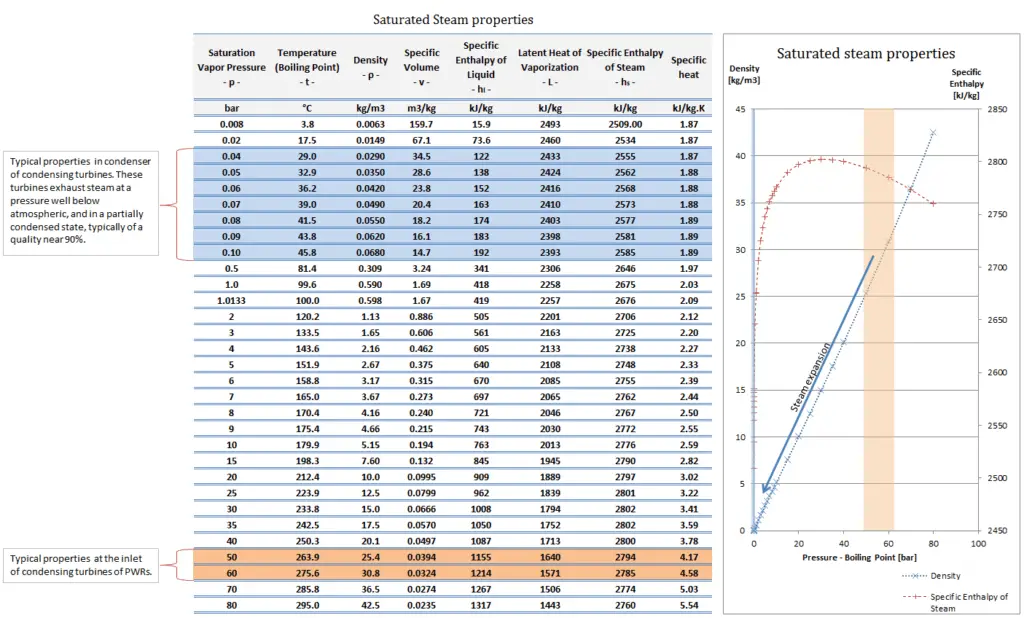
On the other hand condensing steam turbines (at nuclear power plants) exhaust steam at a pressure well below atmospheric (e.g. at 0.08 bar or 8 kPa or 1.16 psia) and in a partially condensed state. In relative units it is a negative gauge pressure of about – 0.92 bar, – 92 kPa, or – 13.54 psig.
Typical Pressures in Engineering – Examples
The pascal (Pa) as a unit of pressure measurement is widely used throughout the world and has largely replaced the pounds per square inch (psi) unit, except in some countries that still use the Imperial measurement system, including the United States. For most engineering problems pascal (Pa) is fairly small unit, so it is convenient to work with multiples of the pascal: the kPa, the MPa, or the bar. Following list summarizes a few examples:
- Typically most of nuclear power plants operates multi-stage condensing steam turbines. These turbines exhaust steam at a pressure well below atmospheric (e.g. at 0.08 bar or 8 kPa or 1.16 psia) and in a partially condensed state. In relative units it is a negative gauge pressure of about – 0.92 bar, – 92 kPa, or – 13.54 psig.
- The Standard Atmospheric Pressure approximates to the average pressure at sea-level at the latitude 45° N. The Standard Atmospheric Pressure is defined at sea-level at 273oK (0oC) and is:
- 101325 Pa
- 1.01325 bar
- 14.696 psi
- 760 mmHg
- 760 torr
- Car tire overpressure is about 2.5 bar, 0.25 MPa, or 36 psig.
- Steam locomotive fire tube boiler: 150–250 psig
- A high-pressure stage of condensing steam turbine at nuclear power plant operates at steady state with inlet conditions of 6 MPa (60 bar, or 870 psig), t = 275.6°C, x = 1
- A boiling water reactor is cooled and moderated by water like a PWR, but at a lower pressure (e.g. 7MPa, 70 bar, or 1015 psig), which allows the water to boil inside the pressure vessel producing the steam that runs the turbines.
- Pressurized water reactors are cooled and moderated by high-pressure liquid water (e.g. 16MPa, 160 bar, or 2320 psig). At this pressure water boils at approximately 350°C (662°F), which provides subcooling margin of about 25°C.
- The supercritical water reactor (SCWR) is operated at supercritical pressure. The term supercritical in this context refers to the thermodynamic critical point of water (TCR = 374 °C; pCR = 22.1 MPa)
- Common rail direct fuel injection: On diesel engines, it features a high-pressure (over 1 000 bar or 100 MPa or 14500 psi) fuel rail.
What is Temperature
In physics and in everyday life a temperature is an objective comparative measurement of hot or cold based on our sense of touch. A body that feels hot usually has a higher temperature than a similar body that feels cold. But this definition is not a simple matter. For example, a metal rod feels colder than a plastic rod at room temperature simply because metals are generally better at conducting heat away from the skin as are plastics. Simply hotness may be represented abstractly and therefore it is necessary to have an objective way of measuring temperature. It is one of basic thermodynamic properties.
Thermal Equilibrium
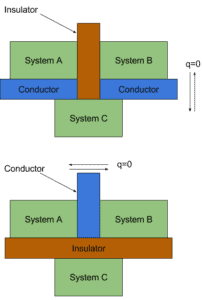
A particularly important concept is thermodynamic equilibrium. In general, when two objects are brought into thermal contact, heat will flow between them until they come into equilibrium with each other. When a temperature difference does exist heat flows spontaneously from the warmer system to the colder system. Heat transfer occurs by conduction or by thermal radiation. When the flow of heat stops, they are said to be at the same temperature. They are then said to be in thermal equilibrium.
For example, you leave a thermometer in a cup of coffee. As the two objects interact, the thermometer becomes hotter and the coffee cools off a little until they come into thermal equilibrium. Two objects are defined to be in thermal equilibrium if, when placed in thermal contact, no net energy flows from one to the other, and their temperatures don’t change. We may postulate:
When the two objects are in thermal equilibrium, their temperatures are equal.
This is a subject of a law that is called the “zeroth law of thermodynamics”.
We can discover an important property of thermal equilibrium by considering three systems. A, B, and C, that initially are not in thermal equilibrium. We separate systems A and B with an adiabatic wall (ideal insulating material), but we let system C interact with both systems A and B. We wait until thermal equilibrium is reached; then A and B are each in thermal equilibrium with C. But are they in thermal equilibrium with each other?
According to many experiments, there will be no net energy flow between A and B. This is an experimental evidence of the following statement:
If two systems are both in thermal equilibrium with a third then they are in thermal equilibrium with each other.
This statement is known as the zeroth law of thermodynamics. It has this unusual name because it was not until after the great first and second laws of thermodynamics were worked out that scientists realized that this apparently obvious postulate needed to be stated first.
This law provides a definition and method of defining temperatures, perhaps the most important intensive property of a system when dealing with thermal energy conversion problems. Temperature is a property of a system that determines whether the system will be in thermal equilibrium with other systems. When two systems are in thermal equilibrium, their temperatures are, by definition, equal, and no net thermal energy will be exchanged between them. Thus the importance of the zeroth law is that it allows a useful definition of temperature.
For example, most materials expand when their temperature is increased. This property is very important in all of the science and engineering, even in nuclear engineering. The thermodynamic efficiency of power plants changes with temperature of inlet steam or even with outside temperature. At higher temperatures, solids such as steel glow orange or even white depending on temperature. The white light from an ordinary incandescent lightbulb comes from an extremely hot tungsten wire. It can be seen temperature is one of the fundamental characteristics that describes matter and influences matter behaviour.
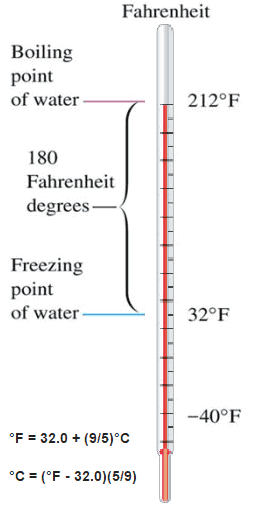
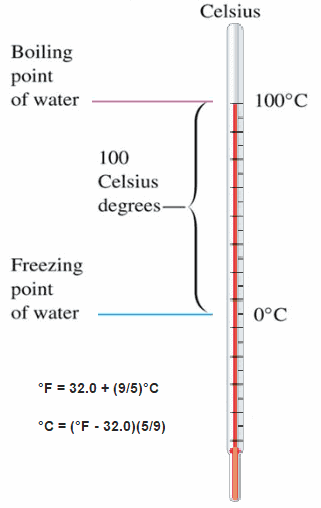
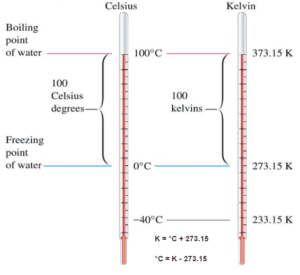
Temperature Scales
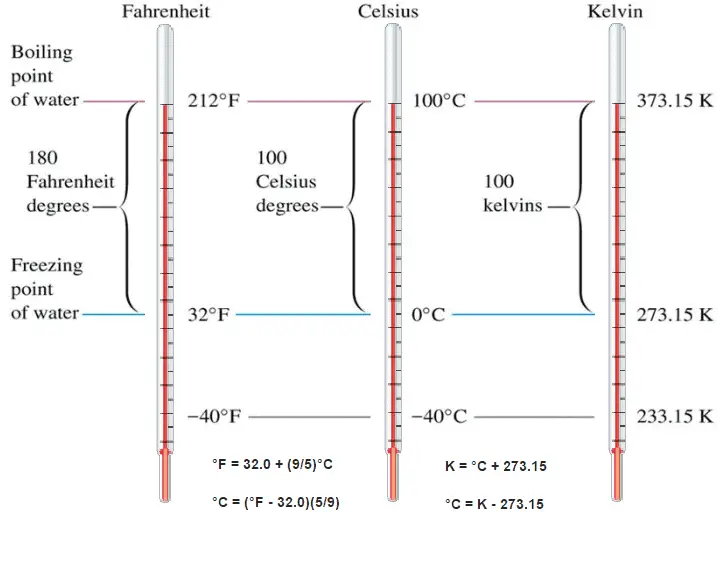
These numbers are arbitrary, and historically many different schemes have been used. For example, this was done by defining some physical occurrences at given temperatures—such as the freezing and boiling points of water — and defining them as 0 and 100 respectively.
There are several scales and units exist for measuring temperature. The most common are:
- Celsius (denoted °C),
- Fahrenheit (denoted °F),
- Kelvin (denoted K; especially in science).
The Celsius scale and the Fahrenheit scale are based on a specification of the number of increments between the freezing point and boiling point of water at standard atmospheric pressure. The Celsius scale has 100 units between these points, and the Fahrenheit scale has 180 units, where each units represents 1°C or 1 °F respectively. The zero points on the scales are arbitrary.
Fahrenheit scale is based on two points:
- The lower defining point, 0 °F, was established as the temperature of a solution of brine made from equal parts of ice and salt.
- The upper defining point, 96 °F, was established as the average human body temperature (96 °F, about 2.6 °F less than the modern value due to a later redefinition of the scale)
The difference in height between the two points would then be marked off in 180 divisions with each division representing 1 °F. The scale is today usually defined by two fixed points: the temperature at which water freezes into ice is defined as 32 °F, and the boiling point of water is defined to be 212 °F.
Temperature Conversion – Fahrenheit – Celsius
To convert from a Fahrenheit temperature to a Celsius temperature we have to subtract 32 degrees from the Fahrenheit reading to get to the zero point on the Celsius scale and then adjust for the different size degrees. The ratio of the size of the degrees is 5/9 so that the relationship between the scales is represented by the following equations:
°F = 32.0 + (9/5)°C
°C = (°F – 32.0)(5/9)
About 20 years after Fahrenheit proposed its temperature scale for thermometer, Swedish professor Anders Celsius defined a better scale for measuring temperature. He proposed using the boiling point of water as 100° C and the freezing point of water as 0° C. Water was chosen as the reference material because it was always available in most laboratories around the world.
Celsius temperature scale is also called centigrade temperature scale because of the 100-degree interval between the defined points. The Celsius temperature for a state colder than freezing water is a negative number. The Celsius scale is used, both in everyday life and in science and industry, almost everywhere in the world.
Absolute zero, the lowest temperature possible, is defined as being precisely 0 K and −273.15 °C. The temperature of the triple point of water is defined as precisely 273.16 K and 0.01 °C. This definition fixes the magnitude of both the degree Celsius and the kelvin as precisely 1 part in 273.16 of the difference between absolute zero and the triple point of water.
It must be added, by international agreement the unit “degree Celsius” and the Celsius scale are currently defined by two different points: absolute zero, and the triple point of water (instead of boiling and freezing points). This definition also precisely relates the Celsius scale to the Kelvin scale, which defines the SI base unit of thermodynamic temperature.
Temperature Conversion – Fahrenheit – Celsius
To convert from a Fahrenheit temperature to a Celsius temperature we have to subtract 32 degrees from the Fahrenheit reading to get to the zero point on the Celsius scale and then adjust for the different size degrees. The ratio of the size of the degrees is 5/9 so that the relationship between the scales is represented by the following equations:
°F = 32.0 + (9/5)°C
°C = (°F – 32.0)(5/9)
Kelvin temperature scale is the base unit of thermodynamic temperature measurement in the International System (SI) of measurement. The Kelvin scale was determined based on the Celsius scale, but with a starting point at absolute zero. Temperatures in the Kelvin scale are 273 degrees less than in the Celsius scale. The kelvin is defined as the fraction 1⁄273.16 of the thermodynamic temperature of the triple point of water. By international agreement, the triple point of water has been assigned a value of 273.16 K (0.01 °C; 32.02 °F) and a partial vapor pressure of 611.66 pascals (6.1166 mbar; 0.0060366 atm). In other words, it is defined such that the triple point of water is exactly 273.16 K.
Note that the unit on the absolute scale is Kelvins, not degrees Kelvin. It was named in honor of Lord Kelvin who had a great deal to do with the development of temperature measurement and thermodynamics.
The absolute temperature scale that corresponds to the Celsius scale is called the Kelvin (K) scale, and the absolute scale that corresponds to the Fahrenheit scale is called the Rankine (R) scale. The zero points on both absolute scales represent the same physical state. The relationships between the absolute and relative temperature scales are shown in the following equations.
Kelvin – Celsius
K = °C + 273.15
°C = K – 273.15
Rankine – Fahrenheit
R = °F + 460
°F = R – 460
Absolute Zero
Such a scale has as its zero point. The coldest theoretical temperature is called absolute zero, at which the thermal motion of atoms and molecules reaches its minimum. This is a state at which the enthalpy and entropy of a cooled ideal gas reaches its minimum value, taken as 0. Classically, this would be a state of motionlessness, but quantum uncertainty dictates that the particles still possess a finite zero-point energy. Absolute zero is denoted as 0 K on the Kelvin scale, −273.15 °C on the Celsius scale, and −459.67 °F on the Fahrenheit scale.
Absolute Zero and Third Law of Thermodynamics
Third law of thermodynamics states:
The entropy of a system approaches a constant value as the temperature approaches absolute zero.
Based on empirical evidence, this law states that the entropy of a pure crystalline substance is zero at the absolute zero of temperature, 0 K and that it is impossible by means of any process, no matter how idealized, to reduce the temperature of a system to absolute zero in a finite number of steps. This allows us to define a zero point for the thermal energy of a body.
We hope, this article, Thermodynamic Property, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.