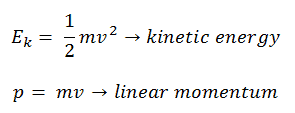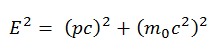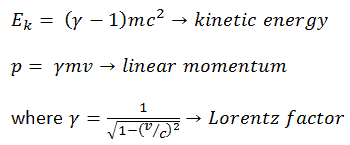Kinetic Energy Formula
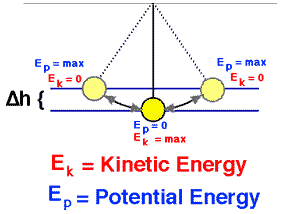
The kinetic energy, K, is defined as the energy stored in an object because of its motion. An object in motion has the ability to do work and thus can be said to have energy. It is called kinetic energy, from the Greek word kinetikos, meaning “motion.”
The kinetic energy depends on the speed of an object and is the ability of a moving object to do work on other objects when it collides with them. On the other hand, the kinetic energy of an object represents the amount of energy required to increase the velocity of the object from rest (v = 0) to its final velocity. The kinetic energy also depends linearly on the mass, which is a numerical measure of object’s inertia and the measure of an object’s resistance to acceleration when a force is applied.
Kinetic Energy Formula:
K = ½ mv2
It is the translational kinetic energy of the object. It must be added, it is called the “translational” kinetic energy to distinguish it from rotational kinetic energy.
Relativistic vs. Non-relativistic Kinetic Energy Formula
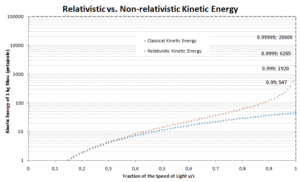
The previous relationship between work and kinetic energy are based on Newton’s laws of motion. When we generalize these laws according to the principle of relativity, we need a corresponding generalization of the equation for kinetic energy. If an object’s speed is close to the speed of light, it is necessary to use relativistic mechanics to calculate its kinetic energy.
In classical mechanics, kinetic energy and momentum are expressed as:
Derivation of its relativistic relationships is based on the relativistic energy-momentum relation:
It can be derived, the relativistic kinetic energy and the relativistic momentum are:
The first term (ɣmc2) of the relativistic kinetic energy increases with the speed v of the particle. The second term (mc2) is constant; it is called the rest energy (rest mass) of the particle, and represents a form of energy that a particle has even when at zero velocity. As velocity of an object approaches the speed of light, the kinetic energy approaches infinity. It is caused by the Lorentz factor, which approaches infinity for v → c. Therefore the speed of light cannot be reached by any massive particles.
The first term (ɣmc2) is known as the total energy E of the particle, because it equals the rest energy plus the kinetic energy:
E = K + mc2
For a particle at rest, i.e. K is zero, so the total energy is its rest energy:
E = mc2
This is one of the striking results of Einstein’s theory of relativity is that mass and energy are equivalent and convertible one into the other. Equivalence of the mass and energy is described by Einstein’s famous formula E = mc2. This result have been experimentally confirmed countless times in nuclear and elementary particle physics. For example, see Positron-electron Pair Production or Conservation of Energy in Nuclear Reactions.
See also: Relativistic Mass
We hope, this article, Kinetic Energy Formula – Equation, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.