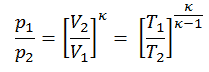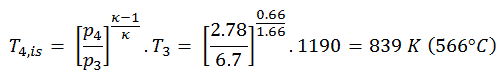Facebook Instagram Youtube Twitter

What is Example of Isentropic Process – Definition

by
Example of Isentropic Process. Assume an isentropic expansion of helium (3 → 4) in a gas turbine. Use the ideal gas law to calculate outlet temperature. Thermal Engineering

Example: Isentropic Expansion in Gas Turbine

P-V diagram - isentropic process
P-V diagram of an isentropic expansion of helium (3 → 4) in a gas turbine.

Assume an isentropic expansion of helium (3 → 4) in a gas turbine. Since helium behaves almost as an ideal gas, use the ideal gas law to calculate outlet temperature of the gas (T4,is). In this turbines the high-pressure stage receives gas (point 3 at the figure; p3 = 6.7 MPa; T3 = 1190 K (917°C)) from a heat exchanger and exhaust it to another heat exchanger, where the outlet pressure is p4 = 2.78 MPa (point 4).

Solution:

The outlet temperature of the gas, T4,is, can be calculated using p, V, T Relation for isentropic process (reversible adiabatic process):

p,V,T relation - isentropic process

In this equation the factor for helium is equal to κ=cp/cv=1.66. From the previous equation follows that the outlet temperature of the gas, T4,is, is:

isentropic process - example

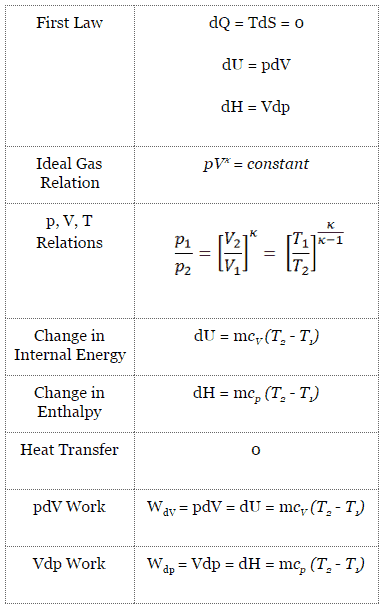
Isentropic Process - characteristics
Table of main characteristics

See also: First Law of Thermodynamics

See also: Ideal Gas Law

See also: What is Enthalpy

Example: Isentropic Expansion in Gas Turbine

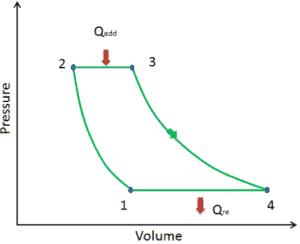
first law - example - brayton cycle
Ideal Brayton cycle consist of four thermodynamic processes. Two isentropic processes and two isobaric processes.

Let assume the ideal Brayton cycle that describes the workings of a constant pressure heat engine. Modern gas turbine engines and airbreathing jet engines also follow the Brayton cycle.

Ideal Brayton cycle consist of four thermodynamic processes. Two isentropic processes and two isobaric processes.

  1. isentropic compression – ambient air is drawn into the compressor, where it is pressurized (1 → 2). The work required for the compressor is given by WC = H2 – H1.
  2. isobaric heat addition – the compressed air then runs through a combustion chamber, where fuel is burned and air or another medium is heated (2 → 3). It is a constant-pressure process, since the chamber is open to flow in and out. The net heat added is given by Qadd = H3 – H2
  3. isentropic expansion – the heated, pressurized air then expands on turbine, gives up its energy. The work done by turbine is given by WT = H4 – H3
  4. isobaric heat rejection – the residual heat must be rejected in order to close the cycle. The net heat rejected is given by Qre = H4 – H1

As can be seen, we can describe and calculate (e.g. thermal efficiency) such cycles (similarly for Rankine cycle) using enthalpies.

See also: Thermal Efficiency of Brayton Cycle

 
References:
Nuclear and Reactor Physics:
  1. J. R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).
  2. J. R. Lamarsh, A. J. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 0-201-82498-1.
  3. W. M. Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1.
  4. Glasstone, Sesonske. Nuclear Reactor Engineering: Reactor Systems Engineering, Springer; 4th edition, 1994, ISBN: 978-0412985317
  5. W.S.C. Williams. Nuclear and Particle Physics. Clarendon Press; 1 edition, 1991, ISBN: 978-0198520467
  6. Kenneth S. Krane. Introductory Nuclear Physics, 3rd Edition, Wiley, 1987, ISBN: 978-0471805533
  7. G.R.Keepin. Physics of Nuclear Kinetics. Addison-Wesley Pub. Co; 1st edition, 1965
  8. Robert Reed Burn, Introduction to Nuclear Reactor Operation, 1988.
  9. U.S. Department of Energy, Nuclear Physics and Reactor Theory. DOE Fundamentals Handbook, Volume 1 and 2. January 1993.

Advanced Reactor Physics:

  1. K. O. Ott, W. A. Bezella, Introductory Nuclear Reactor Statics, American Nuclear Society, Revised edition (1989), 1989, ISBN: 0-894-48033-2.
  2. K. O. Ott, R. J. Neuhold, Introductory Nuclear Reactor Dynamics, American Nuclear Society, 1985, ISBN: 0-894-48029-4.
  3. D. L. Hetrick, Dynamics of Nuclear Reactors, American Nuclear Society, 1993, ISBN: 0-894-48453-2.
  4. E. E. Lewis, W. F. Miller, Computational Methods of Neutron Transport, American Nuclear Society, 1993, ISBN: 0-894-48452-4.

See also:

Isentropic Process

We hope, this article, Example of Isentropic Process, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.

Related Posts