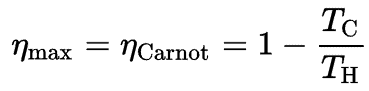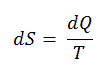Facebook Instagram Youtube Twitter

What is Statement of the Second Law – Definition

by
Statements of the Second Law. The second law of thermodynamics may be expressed in many specific ways. Each statement expresses the same law. There are three that are often encountered

Various Statements of the Second Law of Thermodynamics

The second law of thermodynamics may be expressed in many specific ways. Each statement expresses the same law. Listed below are three that are often encountered.

Before these statements, we have to remind the work of a French engineer and physicist, Nicolas Léonard Sadi Carnot advanced the study of the second law by forming a principle (also called Carnot’s rule) that specifies limits on the maximum efficiency any heat engine can obtain.

 
Carnot's Principle
In 1824, a French engineer and physicist, Nicolas Léonard Sadi Carnot advanced the study of the second law by forming a principle (also called Carnot’s rule) that specifies limits on the maximum efficiency any heat engine can obtain. In short, this principle states that the efficiency of a thermodynamic cycle depends solely on the difference between the hot and cold temperature reservoirs.

Carnot’s principle states:

  1. No engine can be more efficient than a reversible engine (a Carnot heat engine) operating between the same high temperature and low temperature reservoirs.
  2. The efficiencies of all reversible engines (Carnot heat engines) operating between the same constant temperature reservoirs are the same, regardless of the working substance employed or the operation details.

Carnot Efficiency

The formula for this maximum efficiency is:

Carnot Efficiency Formula

where:

  • is the efficiency of Carnot cycle, i.e. it is the ratio = W/QH of the work done by the engine to the heat energy entering the system from the hot reservoir.
  • TC is the absolute temperature (Kelvins) of the cold reservoir,
  • TH is the absolute temperature (Kelvins) of the hot reservoir.
Example: Carnot efficiency for coal-fired power plant
In a modern coal-fired power plant, the temperature of high pressure steam (Thot) would be about 400°C (673K) and Tcold, the cooling tower water temperature, would be about 20°C (293K). For this type of power plant the maximum (ideal) efficiency will be:

= 1 – Tcold/Thot = 1 – 293/673 = 56%

It must be added, this is an idealized efficiency. The Carnot efficiency is valid for reversible processes. These processes cannot be achieved in real cycles of power plants. The Carnot efficiency dictates that higher efficiencies can be attained by increasing the temperature of the steam. This feature is valid also for real thermodynamic cycles. But this requires an increase in pressures inside boilers or steam generators. However, metallurgical considerations place an upper limits on such pressures. Sub-critical fossil fuel power plants, that are operated under critical pressure (i.e. lower than 22.1 MPa), can achieve 36–40% efficiency. Supercritical designs, that are operated at supercritical pressure (i.e. greater than 22.1 MPa),  have efficiencies around 43%. Most efficient and also very complex coal-fired power plants that are operated at “ultra critical” pressures (i.e. around 30 MPa) and use multiple stage reheat reach about 48% efficiency.

See also: Supercritical Reactor

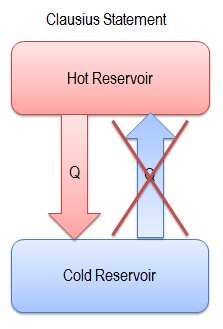
Clausius Statement of the Second Law

Clausius Statement of the Second LawOne of the earliest statements of the Second Law of Thermodynamics was made by R. Clausius in 1850. He stated the following.

“It is impossible to construct a device which operates on a cycle and whose sole effect is the transfer of heat from a cooler body to a hotter body”.

Heat cannot spontaneously flow from cold system to hot system without external work being performed on the system. This is exactly what refrigerators and heat pumps accomplish. In a refrigerator, heat flows from cold to hot, but only when forced by an external work, refrigerators are driven by electric motors requiring work from their surroundings to operate.

The Clausius and the Kelvin-Planck statements have been shown to be equivalent.

Kelvin-Planck Statement of the Second Law

Kelvin-Planck Statement of the Second Law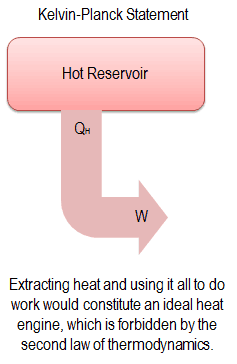“It is impossible to construct a device which operates on a cycle and produces no other effect than the production of work and the transfer of heat from a single body”.

This statement operates with the term “thermal reservoir” or “single reservoir”.  A reservoir is a large object, in which the temperature remains constant while energy is extracted. Such a system can be approximated in a number of ways—by the earth’s atmosphere, large bodies of water like lakes, oceans and so on.

The Kelvin–Planck statement does not exclude the existence of a system, that develops a net amount of work from a heat transfer extracted from a thermal reservoir. According to this statement, a system undergoing a cycle cannot develop a positive net amount of work from a heat transfer extracted from a thermal reservoir.

Entropy and the Second Law

T-s diagram of a thermodynamic cycle
T-s diagram of Rankine Cycle

One consequence of the second law of thermodynamics is the development of the physical property of matter, that is known as the entropy (S). The change in this property is used to determine the direction in which a given process will proceed. Entropy quantifies the energy of a substance that is no longer available to perform useful work. This relates to the second law since the second law predicts that not all heat provided to a cycle can be transformed into an equal amount of work, some heat rejection must take place.

See also: Entropy

According to Clausius, the entropy was defined via the change in entropy S of a system. The change in entropy S, when an amount of heat Q is added to it by a reversible process at constant temperature, is given by:

Entropy- Equation

Here Q is the energy transferred as heat to or from the system during the process, and T is the temperature of the system in kelvins during the process. The SI unit for entropy is J/K.

The second law of thermodynamics can also be expressed as ∆S≥0 for a closed cycle.

In words:

The entropy of any isolated system never decreases. In a natural thermodynamic process, the sum of the entropies of the interacting thermodynamic systems increases.

∆S≥0

Because entropy tells so much about the usefulness of an amount of heat transferred in performing work, the steam tables include values of specific entropy (s = S/m) as part of the information tabulated.

 
References:
Nuclear and Reactor Physics:
  1. J. R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).
  2. J. R. Lamarsh, A. J. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 0-201-82498-1.
  3. W. M. Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1.
  4. Glasstone, Sesonske. Nuclear Reactor Engineering: Reactor Systems Engineering, Springer; 4th edition, 1994, ISBN: 978-0412985317
  5. W.S.C. Williams. Nuclear and Particle Physics. Clarendon Press; 1 edition, 1991, ISBN: 978-0198520467
  6. Kenneth S. Krane. Introductory Nuclear Physics, 3rd Edition, Wiley, 1987, ISBN: 978-0471805533
  7. G.R.Keepin. Physics of Nuclear Kinetics. Addison-Wesley Pub. Co; 1st edition, 1965
  8. Robert Reed Burn, Introduction to Nuclear Reactor Operation, 1988.
  9. U.S. Department of Energy, Nuclear Physics and Reactor Theory. DOE Fundamentals Handbook, Volume 1 and 2. January 1993.

Advanced Reactor Physics:

  1. K. O. Ott, W. A. Bezella, Introductory Nuclear Reactor Statics, American Nuclear Society, Revised edition (1989), 1989, ISBN: 0-894-48033-2.
  2. K. O. Ott, R. J. Neuhold, Introductory Nuclear Reactor Dynamics, American Nuclear Society, 1985, ISBN: 0-894-48029-4.
  3. D. L. Hetrick, Dynamics of Nuclear Reactors, American Nuclear Society, 1993, ISBN: 0-894-48453-2.
  4. E. E. Lewis, W. F. Miller, Computational Methods of Neutron Transport, American Nuclear Society, 1993, ISBN: 0-894-48452-4.

See also:

Second Law

We hope, this article, Statement of the Second Law, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.

Related Posts