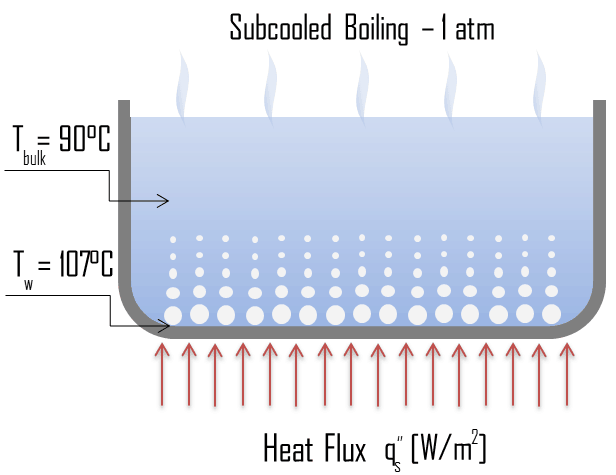
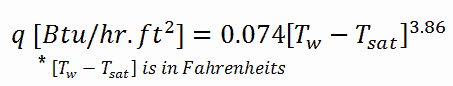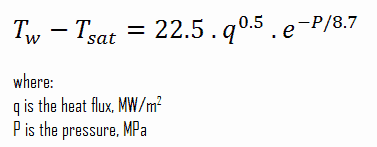Subcooled Boiling
In subcooled boiling, the temperature of most of the liquid is below the saturation temperature and bubbles formed at the surface may condense in the liquid. This condensation (collapsing) produces a sound of frequency ~ 100Hz – 1 KHz. This is why an electric kettle makes the most noise before the water comes to saturated boiling. The term subcooling refers to a liquid existing at a temperature below its normal boiling point.
Source: wikipedia.org CC BY-SA
In preceding chapters, we have discussed convective heat transfer with very important assumption. We have assumed a single-phase convective heat transfer without any phase change. In this chapter we focus on convective heat transfer associated with the change in phase of a fluid. In particular, we consider processes that can occur at a solid–liquid or solid–vapor interface, namely, boiling (liquid-to-vapor phase change) and condensation (vapor-to-liquid phase change).
For these cases latent heat effects associated with the phase change are significant. Latent heat, known also as the enthalpy of vaporization, is the amount of heat added to or removed from a substance to produce a change in phase. This energy breaks down the intermolecular attractive forces, and also must provide the energy necessary to expand the gas (the pΔV work). When latent heat is added, no temperature change occurs.
The enthalpy of vaporization is a function of the pressure at which that transformation takes place.
Latent heat of vaporization – water at 0.1 MPa (atmospheric pressure)
hlg = 2257 kJ/kg
Latent heat of vaporization – water at 3 MPa
hlg = 1795 kJ/kg
Latent heat of vaporization – water at 16 MPa (pressure inside a pressurizer)
hlg = 931 kJ/kg
The heat of vaporization diminishes with increasing pressure, while the boiling point increases. It vanishes completely at a certain point called the critical point. Above the critical point, the liquid and vapor phases are indistinguishable, and the substance is called a supercritical fluid.
The change from the liquid to the vapor state due to boiling is sustained by heat transfer from the solid surface; conversely, condensation of a vapor to the liquid state results in heat transfer to the solid surface. Boiling and condensation differ from other forms of convection in that they depend on the latent heat of vaporization, which is very high for common pressures, therefore large amounts of heat can be transferred during boiling and condensation essentially at constant temperature. Heat transfer coefficients, h, associated with boiling and condensation are typically much higher than those encountered in other forms of convection processes that involve a single phase.
This is due to the fact, even in turbulent flow, there is a stagnant fluid film layer (laminar sublayer), that isolates the surface of the heat exchanger. This stagnant fluid film layer plays crucial role for the convective heat transfer coefficient. It is observed, that the fluid comes to a complete stop at the surface and assumes a zero velocity relative to the surface. This phenomenon is known as the no-slip condition and therefore, at the surface, energy flow occurs purely by conduction. But in the next layers both conduction and diffusion-mass movement in the molecular level or macroscopic level occurs. Due to the mass movement the rate of energy transfer is higher. As was written, nucleate boiling at the surface effectively disrupts this stagnant layer and therefore nucleate boiling significantly increases the ability of a surface to transfer thermal energy to bulk fluid.
Subcooled Boiling in PWRs
Although the earliest core designs assumed that subcooled surface boiling could not be allowed in PWRs, this assumption was soon rejected and two-phase heat transfer is now one of normal operation heat transfer mechanisms also in PWRs. For PWRs at normal operation, there is a compressed liquid water inside the reactor core, loops and steam generators. The pressure is maintained at approximately 16MPa. At this pressure water boils at approximately 350°C(662°F), which gives a subcooling margin (the difference between the pressurizer temperature and the coolant outlet temperature in the reactor core) of 30 °C. Noteworthy, this subcooling margin concerns the bulk temperature, since bulk boiling is in any case prohibited.
Subcooling margin is very important safety parameter of PWRs, since the bulk boiling in the reactor core must be excluded. The basic design of the pressurized water reactor includes such requirement that the coolant (water) in the reactor coolant system must not boil. To achieve this, the coolant in the reactor coolant system is maintained at a pressure sufficiently high that boiling does not occur at the coolant temperatures experienced while the plant is operating or in an analyzed transient.
As was calculated in example, the surface temperature TZr,1 = 325°C ensures, that even subcooled boiling does not occur. Note that, subcooled boiling requires TZr,1 = Tsat. Since the inlet temperatures of the water are usually about 290°C (554°F), it is obvious this example corresponds to the lower part of the core. At higher elevations of the core the bulk temperature may reach up to 330°C. The temperature difference of 29°C causes the subcooled surface boiling may occur (330°C + 29°C > 350°C). On the other hand, nucleate boiling at the surface effectively disrupts the stagnant layer and therefore nucleate boiling significantly increases the ability of a surface to transfer thermal energy to bulk fluid. As a result, the convective heat transfer coefficient significantly increases and therefore at higher elevations, the temperature difference (TZr,1 – Tbulk) significantly decreases.
In case of PWRs, the critical safety issue is named DNB (departure from nucleate boiling), which causes the formation of a local vapor layer, causing a dramatic reduction in heat transfer capability. This phenomenon occurs in the subcooled or low-quality region. The behaviour of the boiling crisis depends on many flow conditions (pressure, temperature, flow rate), but the boiling crisis occurs at a relatively high heat fluxes and appears to be associated with the cloud of bubbles, adjacent to the surface. These bubbles or film of vapor reduces the amount of incoming water. Since this phenomenon deteriorates the heat transfer coefficient and the heat flux remains, heat then accumulates in the fuel rod causing dramatic rise of cladding and fuel temperature.
Nucleate Boiling – Flow Boiling
In flow boiling (or forced convection boiling), fluid flow is forced over a surface by external means such as a pump, as well as by buoyancy effects. Therefore, flow boiling is always accompanied by other convection effects. Conditions depend strongly on geometry, which may involve external flow over heated plates and cylinders or internal (duct) flow. In nuclear reactors, most of boiling regimes are just forced convection boiling. The flow boiling is also classified as either external and internal flow boiling depending on whether the fluid is forced to flow over a heated surface or inside a heated channel.
Internal flow boiling is much more complicated in nature than external flow boiling because there is no free surface for the vapor to escape, and thus both the liquid and the vapor are forced to flow together. The two-phase flow in a tube exhibits different flow boiling regimes, depending on the relative amounts of the liquid and the vapor phases. Therefore internal forced convection boiling is commonly referred to as two-phase flow.
Nucleate Boiling Correlations – Flow Boiling
McAdams Correlation
In fully developed nucleate boiling with saturated coolant, the wall temperature is determined by local heat flux and pressure and is only slightly dependent on the Reynolds number. For subcooled water at absolute pressures between 0.1 – 0.6 MPa, McAdams correlation gives:
Thom Correlation
The Thom correlation is for the flow boiling (subcooled or saturated at pressures up to about 20 MPa) under conditions where the nucleate boiling contribution predominates over forced convection. This correlation is useful for rough estimation of expected temperature difference given the heat flux:
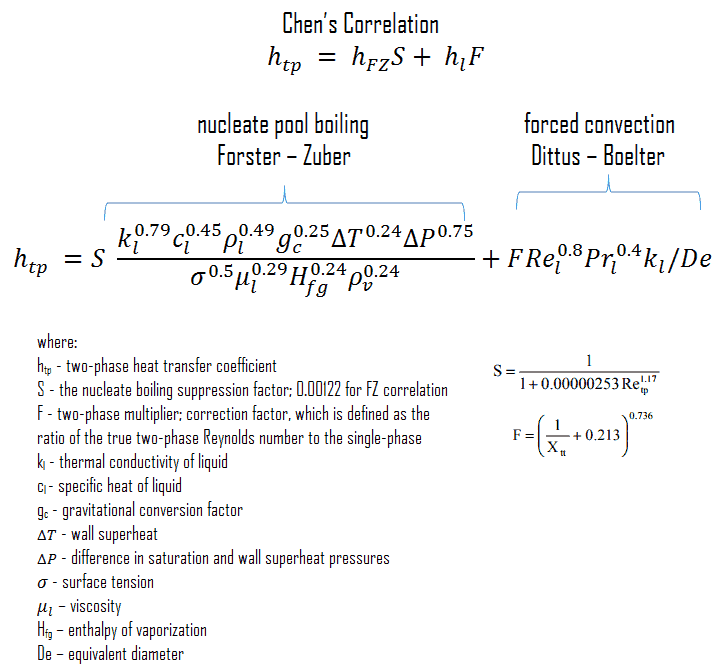
Chen’s Correlation
In 1963, Chen proposed the first flow boiling correlation for evaporation in vertical tubes to attain widespread use. Chen’s correlation includes both the heat transfer coefficients due to nucleate boiling as well as forced convective mechanisms. It must be noted, at higher vapor fractions, the heat transfer coefficient varies strongly with flow rate. The flow velocity in a core can be very high causing very high turbulences. This heat transfer mechanism has been referred to as “forced convection evaporation”. No adequate criteria has been established to determine the transition from nucleate boiling to forced convection vaporization. However, a single correlation that is valid for both nucleate boiling and forced convection vaporization has been developed by Chen for saturated boiling conditions and extended to include subcooled boiling by others. Chen proposed a correlation where the heat transfer coefficient is the sum of a forced convection component and a nucleate boiling component. It must be noted, the nucleate pool boiling correlation of Forster and Zuber (1955) is used to calculate the nucleate boiling heat transfer coefficient, hFZ and the turbulent flow correlation of Dittus-Boelter (1930) is used to calculate the liquid-phase convective heat transfer coefficient, hl.
The nucleate boiling suppression factor, S, is the ratio of the effective superheat to wall superheat. It accounts for decreased boiling heat transfer because the effective superheat across the boundary layer is less than the superheat based on wall temperature. The two-phase multiplier, F, is a function of the Martinelli parameter χtt.
We hope, this article, Subcooled Boiling, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.
