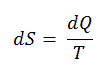Facebook Instagram Youtube Twitter

What is Unit of Entropy – Definition

by
Units of Entropy. The SI unit for entropy is joule per kelvin, J/K. Specific Entropy have units of joule per kilogram times kelvin. Thermal Engineering

Units of Entropy

The SI unit for entropy is J/K. According to Clausius, the entropy was defined via the change in entropy S of a system. The change in entropy S, when an amount of heat Q is added to it by a reversible process at constant temperature, is given by:

Entropy- Equation

Here Q is the energy transferred as heat to or from the system during the process, and T is the temperature of the system in kelvins during the process. If we assume a reversible isothermal process, the total entropy change is given by:

∆S = S2 – S1 = Q/T

In this equation the quotient Q/T is related to the increase in disorder. Higher temperature means greater randomness of motion. At lower temperatures adding heat Q causes a substantial fractional increase in molecular motion and randomness. On the other hand if the substance is already hot, the same quantity of heat Q adds relatively little to the greater molecular motion.

Example: Entropy change in melting ice

Calculate the change in entropy of 1 kg of ice at 0°C, when melted reversibly to water at 0°C.

Since it is an isothermal process, we can use:

∆S = S2 – S1 = Q/T

therefore  the entropy change will be:

∆S = 334 [kJ] / 273.15 [K] = 1.22 [kJ/K]

where 334  kilojoules of heat are required to melt 1 kg of ice (latent heat of fusion = 334 kJ/kg) and this heat is transferred to the system at 0°C (273.15 K).

Specific Entropy

The entropy can be made into an intensive, or specific, variable by dividing by the mass. Engineers use the specific entropy in thermodynamic analysis more than the entropy itself. The specific entropy (s) of a substance is its entropy per unit mass. It equals to the total entropy (S) divided by the total mass (m).

s = S/m

where:

s = specific entropy (J/kg)

S = entropy (J)

m = mass (kg)

T-s diagram of a thermodynamic cycle
T-s diagram of Rankine Cycle

Entropy quantifies the energy of a substance that is no longer available to perform useful work. Because entropy tells so much about the usefulness of an amount of heat transferred in performing work, the steam tables include values of specific entropy (s = S/m) as part of the information tabulated.

In general, specific entropy is a property of a substance, like pressure, temperature, and volume, but it cannot be measured directly. Normally, the entropy of a substance is given with respect to some reference value. For example, the specific entropy of water or steam is given using the reference that the specific entropy of water is zero at 0.01°C and normal atmospheric pressure, where s = 0.00 kJ/kg. The fact that the absolute value of specific entropy is unknown is not a problem, however, because it is the change in specific entropy (∆s) and not the absolute value that is important in practical problems.

 
References:
Nuclear and Reactor Physics:
  1. J. R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).
  2. J. R. Lamarsh, A. J. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 0-201-82498-1.
  3. W. M. Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1.
  4. Glasstone, Sesonske. Nuclear Reactor Engineering: Reactor Systems Engineering, Springer; 4th edition, 1994, ISBN: 978-0412985317
  5. W.S.C. Williams. Nuclear and Particle Physics. Clarendon Press; 1 edition, 1991, ISBN: 978-0198520467
  6. G.R.Keepin. Physics of Nuclear Kinetics. Addison-Wesley Pub. Co; 1st edition, 1965
  7. Robert Reed Burn, Introduction to Nuclear Reactor Operation, 1988.
  8. U.S. Department of Energy, Nuclear Physics and Reactor Theory. DOE Fundamentals Handbook, Volume 1 and 2. January 1993.

Advanced Reactor Physics:

  1. K. O. Ott, W. A. Bezella, Introductory Nuclear Reactor Statics, American Nuclear Society, Revised edition (1989), 1989, ISBN: 0-894-48033-2.
  2. K. O. Ott, R. J. Neuhold, Introductory Nuclear Reactor Dynamics, American Nuclear Society, 1985, ISBN: 0-894-48029-4.
  3. D. L. Hetrick, Dynamics of Nuclear Reactors, American Nuclear Society, 1993, ISBN: 0-894-48453-2.
  4. E. E. Lewis, W. F. Miller, Computational Methods of Neutron Transport, American Nuclear Society, 1993, ISBN: 0-894-48452-4.

See also:

Entropy

We hope, this article, Unit of Entropy, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.

Related Posts