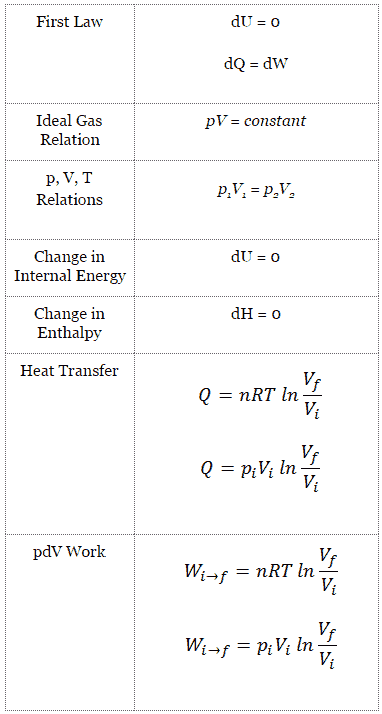Isothermal Process
An isothermal process is a thermodynamic process, in which the temperature of the system remains constant (T = const). The heat transfer into or out of the system typically must happen at such a slow rate in order to continually adjust to the temperature of the reservoir through heat exchange. In each of these states the thermal equilibrium is maintained.
For an ideal gas and a polytropic process, the case n = 1 corresponds to an isothermal (constant-temperature) process. In contrast to adiabatic process , in which n = κ and a system exchanges no heat with its surroundings (Q = 0; ∆T≠0), in an isothermal process there is no change in the internal energy (due to ∆T=0) and therefore ΔU = 0 (for ideal gases) and Q ≠ 0. An adiabatic process is not necessarily an isothermal process, nor is an isothermal process necessarily adiabatic.
In engineering, phase changes, such as evaporation or melting, are isothermal processes when, as is usually the case, they occur at constant pressure and temperature.
Isothermal Expansion – Isothermal Compression
See also: What is an Ideal Gas
 In an ideal gas, molecules have no volume and do not interact. According to the ideal gas law, pressure varies linearly with temperature and quantity, and inversely with volume.
In an ideal gas, molecules have no volume and do not interact. According to the ideal gas law, pressure varies linearly with temperature and quantity, and inversely with volume.
pV = nRT
where:
- p is the absolute pressure of the gas
- n is the amount of substance
- T is the absolute temperature
- V is the volume
- R is the ideal, or universal, gas constant, equal to the product of the Boltzmann constant and the Avogadro constant,
In this equation the symbol R is a constant called the universal gas constant that has the same value for all gases—namely, R = 8.31 J/mol K.
The isothermal process can be expressed with the ideal gas law as:
pV = constant
or
p1V1 = p2V2
On a p-V diagram, the process occurs along a line (called an isotherm) that has the equation p = constant / V.
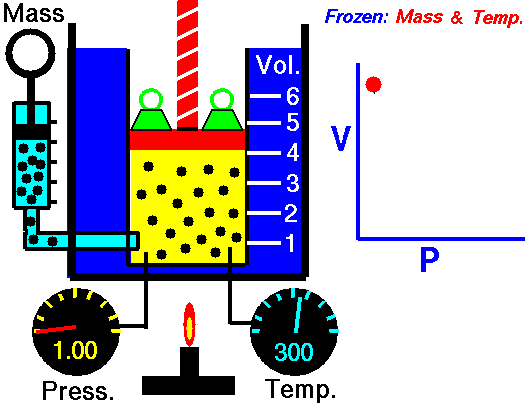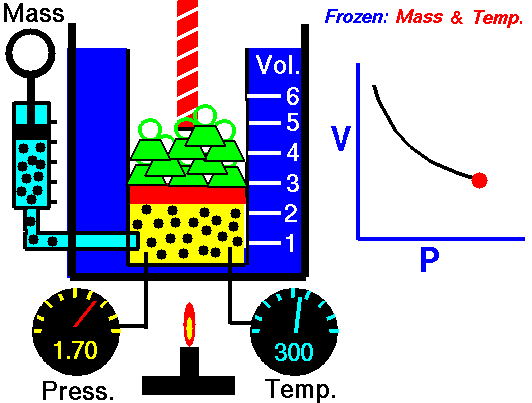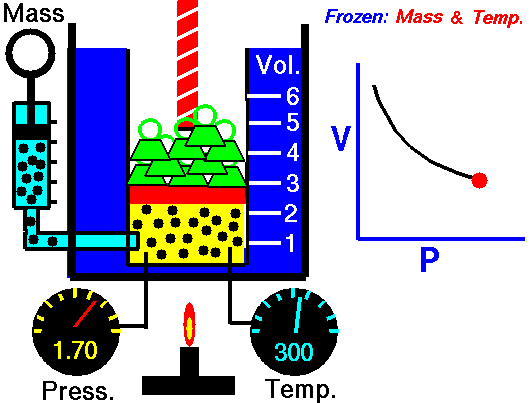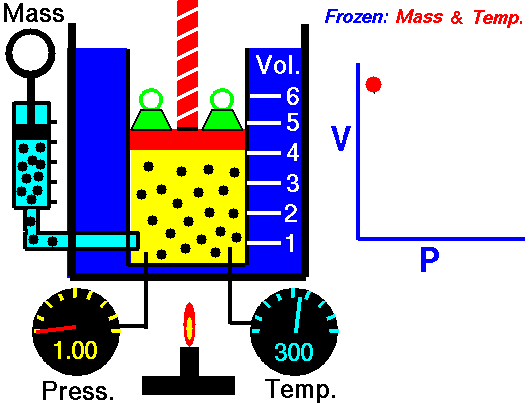
Example of Isothermal Process

Assume an isothermal expansion of helium (i → f) in a frictionless piston (closed system). The gas expansion is propelled by absorption of heat energy Qadd. The gas expands from initial volume of 0.001 m3 and simultaneously the external load of the piston slowly and continuously decreases from 1 MPa to 0.5 MPa. Since helium behaves almost as an ideal gas, use the ideal gas law to calculate final volume of the chamber and then calculate the work done by the system, when the temperature of the gas is equal to 400 K.
Solution:
The final volume of the gas, Vf, can be calculated using p, V, T Relation for isothermal process:
piVi = pfVf ⇒ Vf = piVi / pf = 2 x 0.001 m3 = 0.002 m3
To calculate such processes, we would need to know how pressure varies with volume for the actual process by which the system changes from state i to state f. Since during this process the internal pressure was not constant, the p∆V work done by the piston must be calculated using the following integral:
By convention, a positive value for work indicates that work is done by the system on its surroundings. A negative value indicates that work is done on the system by its surroundings. The pΔV work is equal to the area under the process curve plotted on the pressure-volume diagram.
We hope, this article, Isothermal Expansion – Isothermal Compression, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.