What is Pressure
 Pressure is a measure of the force exerted per unit area on the boundaries of a substance. The standard unit for pressure in the SI system is the Newton per square meter or pascal (Pa). Mathematically:
Pressure is a measure of the force exerted per unit area on the boundaries of a substance. The standard unit for pressure in the SI system is the Newton per square meter or pascal (Pa). Mathematically:
p = F/A
where
- p is the pressure
- F is the normal force
- A is the area of the boundary
Pascal is defined as force of 1N that is exerted on unit area.
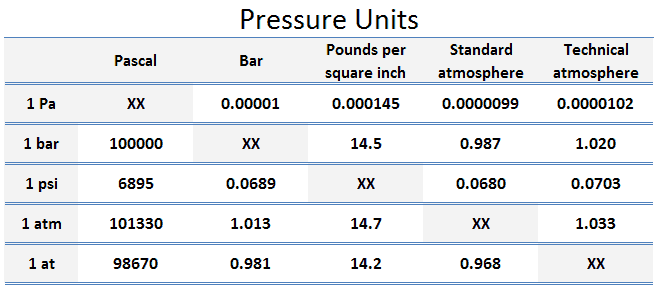
- 1 Pascal = 1 N/m2
- 1 MPa 106 N/m2
- 1 bar 105 N/m2
- 1 kPa 103 N/m2
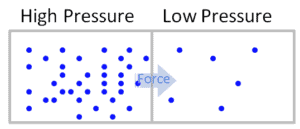
In general, pressure or the force exerted per unit area on the boundaries of a substance is caused by the collisions of the molecules of the substance with the boundaries of the system. As molecules hit the walls, they exert forces that try to push the walls outward. The forces resulting from all of these collisions cause the pressure exerted by a system on its surroundings. Pressure as an intensive variable is constant in a closed system. It really is only relevant in liquid or gaseous systems.
Pressure Scales – Pressure Units
Pascal – Unit of Pressure
As was discussed, the SI unit of pressure and stress is the pascal.
- 1 pascal 1 N/m2 = 1 kg / (m.s2)
Pascal is defined as one newton per square metre. However, for most engineering problems it is fairly small unit, so it is convenient to work with multiples of the pascal: the kPa, the bar, and the MPa.
- 1 MPa 106 N/m2
- 1 bar 105 N/m2
- 1 kPa 103 N/m2
The unit of measurement called standard atmosphere (atm) is defined as:
- 1 atm = 101.33 kPa
The standard atmosphere approximates to the average pressure at sea-level at the latitude 45° N. Note that, there is a difference between the standard atmosphere (atm) and the technical atmosphere (at).
A technical atmosphere is a non-SI unit of pressure equal to one kilogram-force per square centimeter.
- 1 at = 98.67 kPa
Pounds per square inch – psi
The standard unit in the English system is the pound-force per square inch (psi). It is the pressure resulting from a force of one pound-force applied to an area of one square inch.
- 1 psi 1 lbf/in2 = 4.45 N / (0.0254 m)2 ≈ 6895 kg/m2
Therefore, one pound per square inch is approximately 6895 Pa.
The unit of measurement called standard atmosphere (atm) is defined as:
- 1 atm = 14.7 psi
The standard atmosphere approximates to the average pressure at sea-level at the latitude 45° N. Note that, there is a difference between the standard atmosphere (atm) and the technical atmosphere (at).
A technical atmosphere is a non-SI unit of pressure equal to one kilogram-force per square centimeter.
- 1 at = 14.2 psi
Bar – Unit of Pressure
The bar is a metric unit of pressure. It is not part of the International System of Units (SI). The bar is commonly used in the industry and in the meteorology, and an instrument used in meteorology to measure atmospheric pressure is called barometer.
One bar is exactly equal to 100 000 Pa, and is slightly less than the average atmospheric pressure on Earth at sea level (1 bar = 0.9869 atm). Atmospheric pressure is often given in millibars where standard sea level pressure is defined as 1013 mbar, 1.013 bar, or 101.3 (kPa).
Sometimes, “Bar(a)” and “bara” are used to indicate absolute pressures and “bar(g)” and “barg” for gauge pressures.
Absolute vs. Gauge Pressure
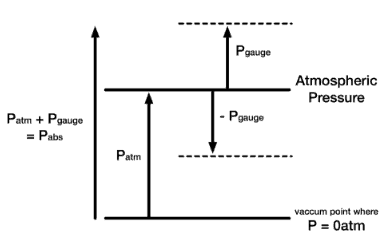
- Absolute Pressure. When pressure is measured relative to a perfect vacuum, it is called absolute pressure (psia). Pounds per square inch absolute (psia) is used to make it clear that the pressure is relative to a vacuum rather than the ambient atmospheric pressure. Since atmospheric pressure at sea level is around 101.3 kPa (14.7 psi), this will be added to any pressure reading made in air at sea level.
- Gauge Pressure. When pressure is measured relative to atmospheric pressure (14.7 psi), it is called gauge pressure (psig). The term gauge pressure is applied when the pressure in the system is greater than the local atmospheric pressure, patm. The latter pressure scale was developed because almost all pressure gauges register zero when open to the atmosphere. Gauge pressures are positive if they are above atmospheric pressure and negative if they are below atmospheric pressure.
pgauge = pabsolute – pabsolute; atm
- Atmospheric Pressure. Atmospheric pressure is the pressure in the surrounding air at – or “close” to – the surface of the earth. The atmospheric pressure varies with temperature and altitude above sea level. The Standard Atmospheric Pressure approximates to the average pressure at sea-level at the latitude 45° N. The Standard Atmospheric Pressure is defined at sea-level at 273oK (0oC) and is:
- 101325 Pa
- 1.01325 bar
- 14.696 psi
- 760 mmHg
- 760 torr
- Negative Gauge Pressure – Vacuum Pressure. When the local atmospheric pressure is greater than the pressure in the system, the term vacuum pressure is used. A perfect vacuum would correspond to absolute zero pressure. It is certainly possible to have a negative gauge pressure, but not possible to have a negative absolute pressure. For instance, an absolute pressure of 80 kPa may be described as a gauge pressure of −21 kPa (i.e., 21 kPa below an atmospheric pressure of 101 kPa).
pvacuum = pabsolute; atm – pabsolute
For example, a car tire pumped up to 2.5 atm (36.75 psig) above local atmospheric pressure (let say 1 atm or 14.7 psia locally), will have an absolute pressure of 2.5 + 1 = 3.5 atm (36.75 + 14.7 = 51.45 psia or 36.75 psig).
On the other hand condensing steam turbines (at nuclear power plants) exhaust steam at a pressure well below atmospheric (e.g. at 0.08 bar or 8 kPa or 1.16 psia) and in a partially condensed state. In relative units it is a negative gauge pressure of about – 0.92 bar, – 92 kPa, or – 13.54 psig.
Ideal Gas Law
Any equation that relates the pressure, temperature, and specific volume of a substance is called an equation of state. The simplest and best-known equation of state for substances in the gas phase is the Ideal Gas equation of state. It was first stated by Émile Clapeyron in 1834 as a combination of the empirical Boyle’s law, Charles’ law and Avogadro’s Law. This equation predicts the p-v-T behavior of a gas quite accurately for dilute or low-pressure gases. In an ideal gas, molecules have no volume and do not interact. According to the ideal gas law, pressure varies linearly with temperature and quantity, and inversely with volume.
pV = nRT
where:
- p is the absolute pressure of the gas
- n is the amount of substance
- T is the absolute temperature
- V is the volume
- R is the ideal, or universal, gas constant, equal to the product of the Boltzmann constant and the Avogadro constant,
In this equation the symbol R is a constant called the universal gas constant that has the same value for all gases—namely, R = 8.31 J/mol K.
The power of the ideal gas law is in its simplicity. When any two of the thermodynamic variables, p, v, and T are given, the third can easily be found. An ideal gas is defined as one in which all collisions between atoms or molecules are perfectly elastic and in which there are no intermolecular attractive forces. An ideal gas can be visualized as a collection of perfectly hard spheres which collide but which otherwise do not interact with each other. In reality, no real gases are like an ideal gas and therefore no real gases follow the ideal gas law or equation completely. At temperatures near a gases boiling point, increases in pressure will cause condensation to take place and drastic decreases in volume. At very high pressures, the intermolecular forces of a gas are significant. However, most gases are in approximate agreement at pressures and temperatures above their boiling point. The ideal gas law is utilized by engineers working with gases because it is simple to use and approximates real gas behavior.
Typical Pressures in Engineering – Examples
The pascal (Pa) as a unit of pressure measurement is widely used throughout the world and has largely replaced the pounds per square inch (psi) unit, except in some countries that still use the Imperial measurement system, including the United States. For most engineering problems pascal (Pa) is fairly small unit, so it is convenient to work with multiples of the pascal: the kPa, the MPa, or the bar. Following list summarizes a few examples:
- Typically most of nuclear power plants operates multi-stage condensing steam turbines. These turbines exhaust steam at a pressure well below atmospheric (e.g. at 0.08 bar or 8 kPa or 1.16 psia) and in a partially condensed state. In relative units it is a negative gauge pressure of about – 0.92 bar, – 92 kPa, or – 13.54 psig.
- The Standard Atmospheric Pressure approximates to the average pressure at sea-level at the latitude 45° N. The Standard Atmospheric Pressure is defined at sea-level at 273oK (0oC) and is:
- 101325 Pa
- 1.01325 bar
- 14.696 psi
- 760 mmHg
- 760 torr
- Car tire overpressure is about 2.5 bar, 0.25 MPa, or 36 psig.
- Steam locomotive fire tube boiler: 150–250 psig
- A high-pressure stage of condensing steam turbine at nuclear power plant operates at steady state with inlet conditions of 6 MPa (60 bar, or 870 psig), t = 275.6°C, x = 1
- A boiling water reactor is cooled and moderated by water like a PWR, but at a lower pressure (e.g. 7MPa, 70 bar, or 1015 psig), which allows the water to boil inside the pressure vessel producing the steam that runs the turbines.
- Pressurized water reactors are cooled and moderated by high-pressure liquid water (e.g. 16MPa, 160 bar, or 2320 psig). At this pressure water boils at approximately 350°C (662°F), which provides subcooling margin of about 25°C.
- The supercritical water reactor (SCWR) is operated at supercritical pressure. The term supercritical in this context refers to the thermodynamic critical point of water (TCR = 374 °C; pCR = 22.1 MPa)
- Common rail direct fuel injection: On diesel engines, it features a high-pressure (over 1 000 bar or 100 MPa or 14500 psi) fuel rail.
Pressure Loss – Fluids
Summary of: Head Loss – Pressure Loss
- Head loss or pressure loss are the reduction in the total head (sum of potential head, velocity head, and pressure head) of a fluid caused by the friction present in the fluid’s motion.
- Head loss and pressure loss represent the same phenomenon – frictional losses in pipe and losses in hydraulic components, but they are expressed in different units.
- Head loss of hydraulic system is divided into two main categories:
- Major Head Loss – due to friction in straight pipes
- Minor Head Loss – due to components as valves, bends…
-
Source: Donebythesecondlaw at the English language Wikipedia, CC BY-SA 3.0,
https://commons.wikimedia.org/w/index.php?curid=4681366Darcy’s equation can be used to calculate major losses.
- A special form of Darcy’s equation can be used to calculate minor losses.
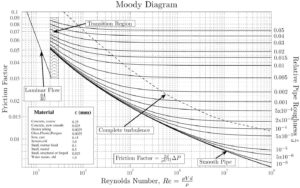
- The friction factor for fluid flow can be determined using a Moody chart.
- The friction factor for laminar flow is independent of roughness of the pipe’s inner surface. f = 64/Re
- The friction factor for turbulent flow depends strongly on the relative roughness. It is determined by the Colebrook equation. It must be noted, at very large Reynolds numbers, the friction factor is independent of the Reynolds number.
- The minor losses are roughly proportional to the square of the flow rate and therefore they can be easy integrated into the Darcy-Weisbach equation through resistance coefficient K.
- As a local pressure loss fluid acceleration in a heated channel can be also considered.
Critical Pressure of Water
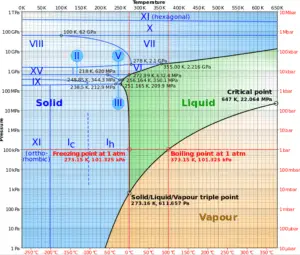
Source: wikipedia.org CC BY-SA
At pressure, that is higher than the critical pressure, water is in special state, that is known as supercritical fluid state. A supercritical fluid is a fluid that is at pressures higher than its thermodynamic critical values. At the critical and supercritical pressures a fluid is considered as a single-phase substance in spite of the fact that all thermophysical properties undergo significant changes within the critical and pseudocritical regions.
For water, the critical parameters are the following:
- Pcr = 22.09 MPa
- Tcr = 374.14 °C (or 647.3 K)
- vcr = 0.003155 m3/kg
- uf = ug = 2014 kJ/kg
- hf = hg = 2084 kJ/kg
- sf = sg =4.406 kJ/kg K
See also: Critical Point of Water
See also: Supercritical Fluid
We hope, this article, Pressure – Physics, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.