What is Formula of First Law of Thermodynamics – Definition

Formulas of First Law of Thermodynamics

The increase in internal energy of a closed system is equal to the heat supplied to the system minus work done by it.

Formula:

∆E_int = Q – W

This is the First Law of Thermodynamics and it is the principle of conservation of energy, meaning that energy can neither be created nor destroyed, but rather transformed into various forms as the fluid within the control volume is being studied.

It is the most important law for analysis of most systems and the one that quantifies how thermal energy is transformed to other forms of energy. It follows, perpetual motion machines of the first kind are impossible.

Differential form:

Differential form:

dE_int = dQ  – dW

The internal energy E_int of a system tends to increase if energy is added as heat Q and tends to decrease if energy is lost as work W done by the system.

First Law in Terms of Enthalpy dH = dQ + Vdp

The enthalpy is defined to be the sum of the internal energy E plus the product of the pressure p and volume V. In many thermodynamic analyses the sum of the internal energy U and the product of pressure p and volume V appears, therefore it is convenient to give the combination a name, enthalpy, and a distinct symbol, H.

H = U + pV

See also: Enthalpy

The first law of thermodynamics in terms of enthalpy show us, why engineers use the enthalpy in thermodynamic cycles (e.g. Brayton cycle or Rankine cycle).

The classical form of the law is the following equation:

dU = dQ  – dW

In this equation dW is equal to dW = pdV and is known as the boundary work.

dH – pdV – Vdp = dQ – pdV

We obtain the law in terms of enthalpy:

dH = dQ + Vdp

or

dH = TdS + Vdp

In this equation the term Vdp is a flow process work. This work,  Vdp, is used for open flow systems like a turbine or a pump in which there is a “dp”, i.e. change in pressure. There are no changes in control volume. As can be seen, this form of the law simplifies the description of energy transfer. At constant pressure, the enthalpy change equals the energy transferred from the environment through heating:

Isobaric process (Vdp = 0):

dH = dQ     →     Q = H₂ – H₁

At constant entropy, i.e. in isentropic process, the enthalpy change equals the flow process work done on or by the system:

Isentropic process (dQ = 0):

dH = Vdp     →     W = H₂ – H₁

It is obvious, it will be very useful in analysis of both thermodynamic cycles used in power engineering, i.e. in Brayton cycle and Rankine cycle.

pΔV Work

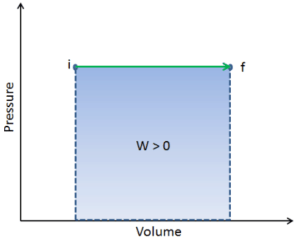
Example:

Consider a frictionless piston that is used to provide a constant pressure of 500 kPa in a cylinder containing steam (superheated steam) of a volume of 2 m³  at 500 K.

Calculate the final temperature, if 3000 kJ of heat is added.

Solution:

Using steam tables we know, that the specific enthalpy of such steam (500 kPa; 500 K) is about 2912 kJ/kg. Since at this condition the steam has density of 2.2 kg/m³, then we know there is about 4.4 kg of steam in the piston at enthalpy of 2912 kJ/kg x 4.4 kg = 12812 kJ.

When we use simply Q = H₂ − H₁, then the resulting enthalpy of steam will be:

H₂ = H₁ + Q = 15812 kJ

From steam tables, such superheated steam (15812/4.4 = 3593 kJ/kg) will have a temperature of 828 K (555°C). Since at this enthalpy the steam have density of 1.31 kg/m³, it is obvious that it has expanded by about 2.2/1.31 = 1.67 (+67%). Therefore the resulting volume is 2 m³ x 1.67 = 3.34 m³ and ∆V = 3.34 m³ – 2 m³ = 1.34 m³.

The p∆V part of enthalpy, i.e. the work done is:

W = p∆V = 500 000 Pa x 1.34 m³ = 670 kJ

———–

See also:

First Law