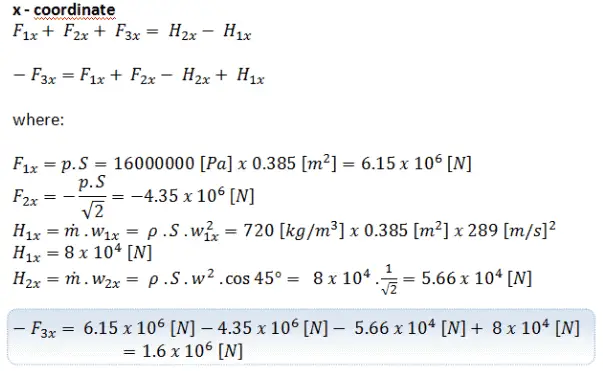Facebook Instagram Youtube Twitter

What is Conservation of Momentum in Fluid Dynamics – Definition

by
In fluid dynamics, the law of conservation of momentum or principle of momentum conservation states that the momentum of an isolated system is a constant. Thermal Engineering

Conservation of Momentum in Fluid Dynamics

In general, the law of conservation of momentum or principle of momentum conservation states that the momentum of an isolated system is a constant. The vector sum of the momenta (momentum is equal to the mass of an object multiplied by its velocity) of all the objects of a system cannot be changed by interactions within the system. In classical mechanics, this law is implied by Newton’s laws.

In fluid dynamics, the analysis of motion is performed in the same way as in solid mechanics – by use of Newton’s laws of motion. As can be seen moving fluids are exerting forces. The lift force on an aircraft is exerted by the air moving over the wing.

A jet of water from a hose exerts a force on whatever it hits. But here it is not clear what mass of moving fluid we should use and therefore it is necessary to use a different form of the equation.

Newton’s 2nd Law can be written:

The rate of change of momentum of a body is equal to the resultant force acting on the body, and takes place in the direction of the force.
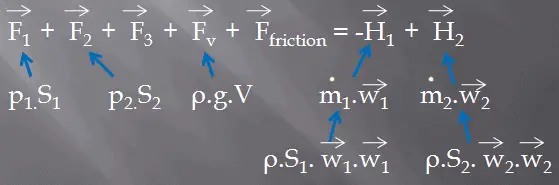
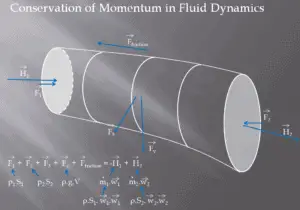Conservation of Momentum - FluidsWe assume fluid to be both steady and incompressible. To determine the rate of change of momentum for a fluid we will consider a streamtube (control volume) as we did for the Bernoulli equation. In this control volume any change in momentum of the fluid within a control volume is due to the action of external forces on the fluid within the volume.

As can be seen from the picture the control volume method can be used to analyze the law of conservation of momentum in fluid. Control volume is an imaginary surface enclosing a volume of interest. The control volume can be fixed or moving, and it can be rigid or deformable. In order to determine all forces acting on the surfaces of the control volume we have to solve the conservation laws in this control volume.

The first conservation equation we have to consider in the control volume is the continuity equation (the law of conservation of matter). In the simplest form it is represented by following equation:

∑ṁin = ∑ṁout

Sum of mass flow rates entering per unit time = Sum of mass flow rates leaving per unit time

The second conservation equation we have to consider in the control volume is the momentum formula.

Momentum Formula

In the simplest form the momentum formula can be represented by following equation:

–
Momentum Formula - fluids

Choosing a Control Volume

A control volume can be selected as any arbitrary volume through which fluid flows. This volume can be static, moving, and even deforming during flow. In order to solve any problem we have to solve basic conservation laws in this volume. It is very important to know all relative flow velocities to the control surface and therefore it is very important to define exactly the boundaries of the control volume during an analysis.

Example: The force acting on a deflector elbow

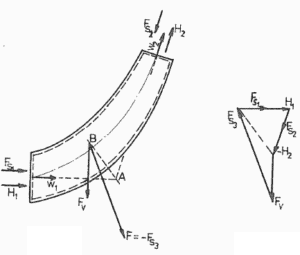Momentum equation - control volumeAn elbow (let say of primary piping) is used to deflect water flow at a velocity of 17 m/s. The piping diameter is equal to 700 mm. The gauge pressure inside the pipe is about 16 MPa at the temperature of 290°C. Fluid is of constant density ⍴ ~ 720 kg/m3 (at 290°C). The angle of the elbow is 45°.

Calculate the force on the wall of a deflector elbow (i.e. calculate vector F3).

Assumptions:

  • The flow is steady.
  • The frictional losses are negligible.
  • The weight of the elbow is negligible.
  • The weight of the water in the elbow is negligible.

We take the elbow as the control volume. The control volume is shown at the picture. The momentum equation is a vector equation so it has three components. We take the x- and z- coordinates as shown and we will solve the problem separately according to these coordinates.

First, let us consider the component in the x-coordinate. The conservation of linear momentum equation becomes:

Momentum equation - x-coordinate

Second, let us consider the component in the y-coordinate. The conservation of linear momentum equation becomes:

Momentum equation - y-coordinate

The final force acting on the wall of a deflector elbow will be:

Momentum equation - resulting force

Example: Water Jet Striking a Stationary Plate

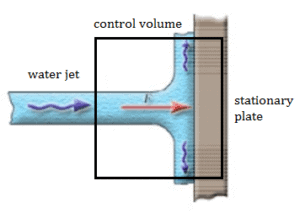Momentum Equation - Water JetA stationary plate (e.g. blade of a watermill) is used to deflect water flow at a velocity of 1 m/s and at an angle of 90°. It occurs at atmospheric pressure and the mass flow rate is equal to Q =1 m3/s.

  1. Calculate the pressure force.
  2. Calculate the body force.
  3. Calculate the total force.
  4. Calculate the resultant force.

Solution

  1. The pressure force is zero as the pressure at both the inlet and the outlets to the control volume are atmospheric.
  2. As the control volume is small we can ignore the body force due to the weight of gravity.
  3. Fx = ρ.Q.(w1x – w2x) = 1000 . 1 . (1 – 0) = 1000 N
    Fy = 0
    F = (1000, 0)
  4. The resultant force on the plane is the same magnitude but in the opposite direction as the total force F (friction and weight are neglected).

The water jet exerts on the plate the force of 1000 N in the x-direction.

 
References:
Reactor Physics and Thermal Hydraulics:
  1. J. R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).
  2. J. R. Lamarsh, A. J. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 0-201-82498-1.
  3. W. M. Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1.
  4. Glasstone, Sesonske. Nuclear Reactor Engineering: Reactor Systems Engineering, Springer; 4th edition, 1994, ISBN: 978-0412985317
  5. Todreas Neil E., Kazimi Mujid S. Nuclear Systems Volume I: Thermal Hydraulic Fundamentals, Second Edition. CRC Press; 2 edition, 2012, ISBN: 978-0415802871
  6. Zohuri B., McDaniel P. Thermodynamics in Nuclear Power Plant Systems. Springer; 2015, ISBN: 978-3-319-13419-2
  7. Moran Michal J., Shapiro Howard N. Fundamentals of Engineering Thermodynamics, Fifth Edition, John Wiley & Sons, 2006, ISBN: 978-0-470-03037-0
  8. Kleinstreuer C. Modern Fluid Dynamics. Springer, 2010, ISBN 978-1-4020-8670-0.
  9. U.S. Department of Energy, THERMODYNAMICS, HEAT TRANSFER, AND FLUID FLOW. DOE Fundamentals Handbook, Volume 1, 2 and 3. June 1992.
  10. White Frank M., Fluid Mechanics, McGraw-Hill Education, 7th edition, February, 2010, ISBN: 978-0077422417

See also:

Fluid Dynamics

We hope, this article, Conservation of Momentum in Fluid Dynamics, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.

Related Posts