What is Enthalpy
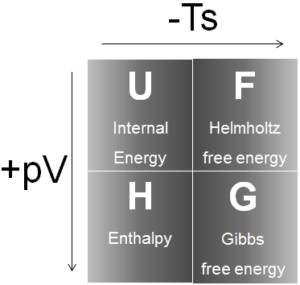
In thermodynamics, the enthalpy is the measure of energy in a thermodynamic system. It is the thermodynamic quantity equivalent to the total heat content of a system. The enthalpy is defined to be the sum of the internal energy E plus the product of the pressure p and volume V. In many thermodynamic analyses the sum of the internal energy U and the product of pressure p and volume V appears, therefore it is convenient to give the combination a name, enthalpy, and a distinct symbol, H.
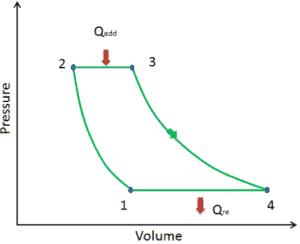
The enthalpy is the preferred expression of system energy changes in many chemical, biological, and physical measurements at constant pressure. It is so useful that it is tabulated in the steam tables along with specific volume and specific internal energy. It is due to the fact, it simplifies the description of energy transfer. At constant pressure, the enthalpy change equals the energy transferred from the environment through heating (Q = H2 – H1) or work other than expansion work. For a variable-pressure process, the difference in enthalpy is not quite as obvious.
Enthalpy in Extensive Units
H = U + pV
Enthalpy is an extensive quantity, it depends on the size of the system, or on the amount of substance it contains. The SI unit of enthalpy is the joule (J). It is the energy contained within the system, excluding the kinetic energy of motion of the system as a whole and the potential energy of the system as a whole due to external force fields. It is the thermodynamic quantity equivalent to the total heat content of a system.
On the other hand, energy can be stored in the chemical bonds between the atoms that make up the molecules. This energy storage on the atomic level includes energy associated with electron orbital states, nuclear spin, and binding forces in the nucleus.
Enthalpy is represented by the symbol H, and the change in enthalpy in a process is H2 – H1.
There are expressions in terms of more familiar variables such as temperature and pressure:
dH = CpdT + V(1-αT)dp
Where Cp is the heat capacity at constant pressure and α is the coefficient of (cubic) thermal expansion. For ideal gas αT = 1 and therefore:
dH = CpdT
Example: Frictionless Piston – Heat – Enthalpy
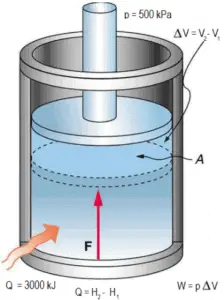
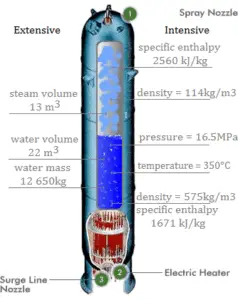
A frictionless piston is used to provide a constant pressure of 500 kPa in a cylinder containing steam (superheated steam) of a volume of 2 m3 at 500 K. Calculate the final temperature, if 3000 kJ of heat is added.
Solution:
Using steam tables we know, that the specific enthalpy of such steam (500 kPa; 500 K) is about 2912 kJ/kg. Since at this condition the steam has density of 2.2 kg/m3, then we know there is about 4.4 kg of steam in the piston at enthalpy of 2912 kJ/kg x 4.4 kg = 12812 kJ.
When we use simply Q = H2 − H1, then the resulting enthalpy of steam will be:
H2 = H1 + Q = 15812 kJ
From steam tables, such superheated steam (15812/4.4 = 3593 kJ/kg) will have a temperature of 828 K (555°C). Since at this enthalpy the steam have density of 1.31 kg/m3, it is obvious that it has expanded by about 2.2/1.31 = 1.67 (+67%). Therefore the resulting volume is 2 m3 x 1.67 = 3.34 m3 and ∆V = 3.34 m3 – 2 m3 = 1.34 m3.
The p∆V part of enthalpy, i.e. the work done is:
W = p∆V = 500 000 Pa x 1.34 m3 = 670 kJ
Enthalpy in Intensive Units – Specific Enthalpy
The enthalpy can be made into an intensive, or specific, variable by dividing by the mass. Engineers use the specific enthalpy in thermodynamic analysis more than the enthalpy itself. The specific enthalpy (h) of a substance is its enthalpy per unit mass. It equals to the total enthalpy (H) divided by the total mass (m).
h = H/m
where:
h = specific enthalpy (J/kg)
H = enthalpy (J)
m = mass (kg)
Note that the enthalpy is the thermodynamic quantity equivalent to the total heat content of a system. The specific enthalpy is equal to the specific internal energy of the system plus the product of pressure and specific volume.
h = u + pv
In general, enthalpy is a property of a substance, like pressure, temperature, and volume, but it cannot be measured directly. Normally, the enthalpy of a substance is given with respect to some reference value. For example, the specific enthalpy of water or steam is given using the reference that the specific enthalpy of water is zero at 0.01°C and normal atmospheric pressure, where hL = 0.00 kJ/kg. The fact that the absolute value of specific enthalpy is unknown is not a problem, however, because it is the change in specific enthalpy (∆h) and not the absolute value that is important in practical problems.
Enthalpy in Chemical Reactions
The enthalpy is widely used also in chemistry. Chemical reactions are determined by the laws of thermodynamics. In thermodynamics, the internal energy of a system is the energy contained within the system, excluding the kinetic energy of motion of the system as a whole and the potential energy of the system as a whole due to external force fields. The enthalpy of a chemical reaction is defined as the enthalpy change observed in a constituent of a thermodynamic system when one mole of substance reacts completely.
Since most of the chemical reactions in laboratory are constant-pressure processes, we can write the change in enthalpy (also known as enthalpy of reaction) for a reaction. The enthalpy of reaction can be positive or negative or zero depending upon whether the heat is gained or lost or no heat is lost or gained. In an endothermic reaction, the products have more stored chemical energy than the reactants. In an exothermic reaction, the opposite is true. The products have less stored chemical energy than the reactants. The excess energy is generally released to the surroundings when the reaction occurs.
In chemical reactions, energy is stored in the chemical bonds between the atoms that make up the molecules. Energy storage on the atomic level includes energy associated with electron orbital states. Whether a chemical reaction absorbs or releases energy, there is no overall change in the amount of energy during the reaction. That’s because of the law of conservation of energy, which states that:
Energy cannot be created or destroyed. Energy may change form during a chemical reaction.
Enthalpy of Vaporization
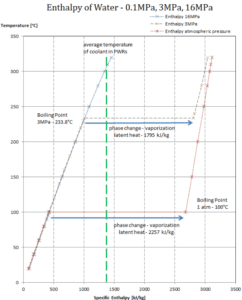
In general, when a material changes phase from solid to liquid, or from liquid to gas a certain amount of energy is involved in this change of phase. In case of liquid to gas phase change, this amount of energy is known as the enthalpy of vaporization, (symbol ∆Hvap; unit: J) also known as the (latent) heat of vaporization or heat of evaporation. Latent heat is the amount of heat added to or removed from a substance to produce a change in phase. This energy breaks down the intermolecular attractive forces, and also must provide the energy necessary to expand the gas (the pΔV work). When latent heat is added, no temperature change occurs. The enthalpy of vaporization is a function of the pressure at which that transformation takes place.
Latent heat of vaporization – water at 0.1 MPa (atmospheric pressure)
hlg = 2257 kJ/kg
Latent heat of vaporization – water at 3 MPa (pressure inside a steam generator)
hlg = 1795 kJ/kg
Latent heat of vaporization – water at 16 MPa (pressure inside a pressurizer)
hlg = 931 kJ/kg
The heat of vaporization diminishes with increasing pressure, while the boiling point increases. It vanishes completely at a certain point called the critical point. Above the critical point, the liquid and vapor phases are indistinguishable, and the substance is called a supercritical fluid.
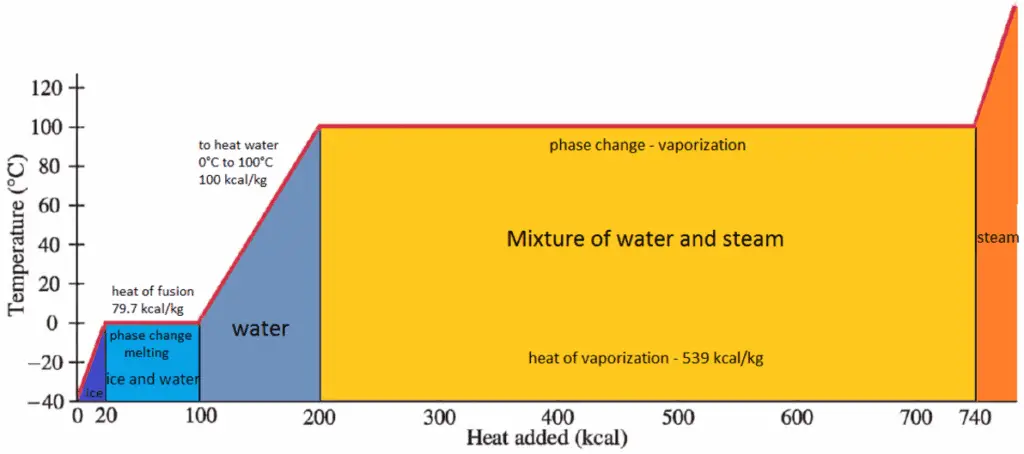
The heat of vaporization is the heat required to completely vaporize a unit of saturated liquid (or condense a unit mass of saturated vapor) and it equal to hlg = hg − hl.
The heat that is necessary to melt (or freeze) a unit mass at the substance at constant pressure is the heat of fusion and is equal to hsl = hl − hs, where hs is the enthalpy of saturated solid and hl is the enthalpy of saturated liquid.
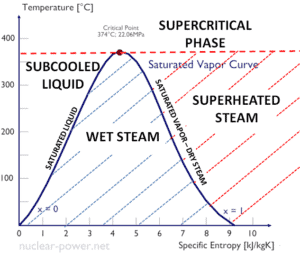
Specific Enthalpy of Wet Steam
 The specific enthalpy of saturated liquid water (x=0) and dry steam (x=1) can be picked from steam tables. In case of wet steam, the actual enthalpy can be calculated with the vapor quality, x, and the specific enthalpies of saturated liquid water and dry steam:
The specific enthalpy of saturated liquid water (x=0) and dry steam (x=1) can be picked from steam tables. In case of wet steam, the actual enthalpy can be calculated with the vapor quality, x, and the specific enthalpies of saturated liquid water and dry steam:
hwet = hs x + (1 – x ) hl
where
hwet = enthalpy of wet steam (J/kg)
hs = enthalpy of “dry” steam (J/kg)
hl = enthalpy of saturated liquid water (J/kg)
As can be seen, wet steam will always have lower enthalpy than dry steam.
Example:
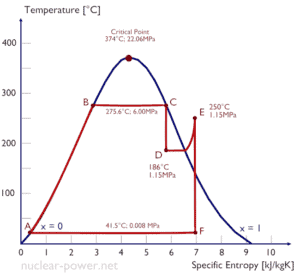
A high-pressure stage of steam turbine operates at steady state with inlet conditions of 6 MPa, t = 275.6°C, x = 1 (point C). Steam leaves this stage of turbine at a pressure of 1.15 MPa, 186°C and x = 0.87 (point D). Calculate the enthalpy difference between these two states.
The enthalpy for the state C can be picked directly from steam tables, whereas the enthalpy for the state D must be calculated using vapor quality:
h1, wet = 2785 kJ/kg
h2, wet = h2,s x + (1 – x ) h2,l = 2782 . 0.87 + (1 – 0.87) . 790 = 2420 + 103 = 2523 kJ/kg
Δh = 262 kJ/kg
We hope, this article, Enthalpy, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.