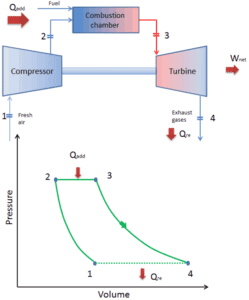
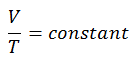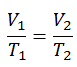What is Brayton Cycle – Processes - Equations

In a closed ideal Brayton cycle, the system executing the cycle undergoes a series of four processes: two isentropic processes alternated with two isobaric processes. Thermal Engineering

Brayton Cycle – Turbine Engine

In 1872, an American engineer, George Bailey Brayton advanced the study of heat engines by patenting a constant pressure internal combustion engine, initially using vaporized gas but later using liquid fuels such as kerosene. This heat engine is known as “Brayton’s Ready Motor”. It means, the original Brayton engine used a piston compressor and piston expander instead of a gas turbine and gas compressor.

Today, modern gas turbine engines and airbreathing jet engines are also a constant-pressure heat engines, therefore we describe their thermodynamics by the Brayton cycle. In general, the Brayton cycle describes the workings of a constant-pressure heat engine.

It is the one of most common thermodynamic cycles that can be found in gas turbine power plants or in airplanes. In contrast to Carnot cycle, the Brayton cycle does not execute isothermal processes, because these must be performed very slowly. In an ideal Brayton cycle, the system executing the cycle undergoes a series of four processes: two isentropic (reversible adiabatic) processes alternated with two isobaric processes.

Since Carnot’s principle states that no engine can be more efficient than a reversible engine (a Carnot heat engine) operating between the same high temperature and low temperature reservoirs, a gas turbine based on the Brayton cycle must have lower efficiency than the Carnot efficiency.

A large single-cycle gas turbine typically produces for example 300 megawatts of electric power and has 35–40% thermal efficiency. Modern Combined Cycle Gas Turbine (CCGT) plants, in which the thermodynamic cycle of consists of two power plant cycles (e.g. the Brayton cycle and the Rankine cycle), can achieve a thermal efficiency of around 55%.

Brayton Cycle – Processes

Brayton Cycle – Processes

In a closed ideal Brayton cycle, the system executing the cycle undergoes a series of four processes: two isentropic (reversible adiabatic) processes alternated with two isobaric processes:

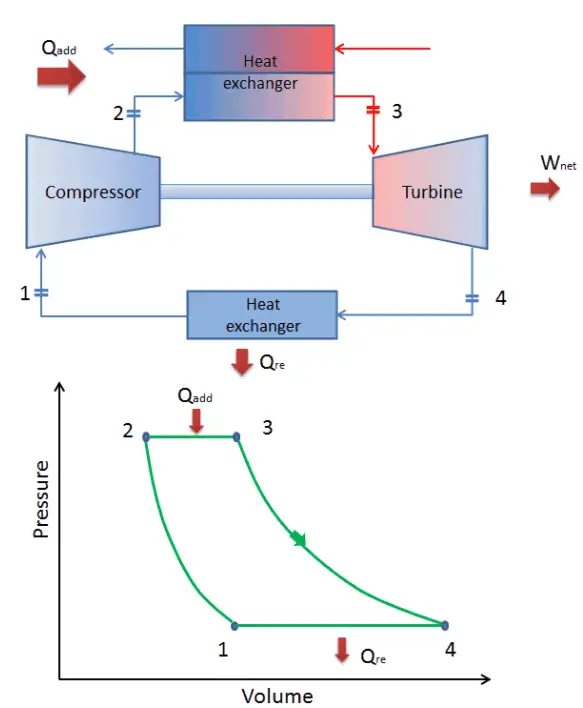
closed Brayton cycle

Isentropic compression (compression in a compressor) – The working gas (e.g. helium) is compressed adiabatically from state 1 to state 2 by the compressor (usually an axial-flow compressor). The surroundings do work on the gas, increasing its internal energy (temperature) and compressing it (increasing its pressure). On the other hand the entropy remains unchanged. The work required for the compressor is given by W_C = H₂ – H₁.
Isobaric heat addition (in a heat exchanger) – In this phase (between state 2 and state 3) there is a constant-pressure heat transfer to the gas from an external source, since the chamber is open to flow in and out. In an open ideal Brayton cycle, the compressed air then runs through a combustion chamber, where fuel is burned and air or another medium is heated (2 → 3). It is a constant-pressure process, since the chamber is open to flow in and out. The net heat added is given by Q_add = H₃– H₂
Isentropic expansion (expansion in a turbine) – The compressed and heated gas expands adiabatically from state 3 to state 4 in a turbine. The gas does work on the surroundings (blades of the turbine) and loses an amount of internal energy equal to the work that leaves the system. The work done by turbine is given by W_T = H₄ – H₃. Again the entropy remains unchanged.
Isobaric heat rejection (in a heat exchanger) – In this phase the cycle completes by a constant-pressure process in which heat is rejected from the gas. The working gas temperature drops from point 4 to point 1. The net heat rejected is given by Q_re = H₄– H₁

During a Brayton cycle, work is done on the gas by the compressor between states 1 and 2 (isentropic compression). Work is done by the gas in the turbine between stages 3 and 4 (isentropic expansion). The difference between the work done by the gas and the work done on the gas is the net work produced by the cycle and it corresponds to the area enclosed by the cycle curve (in pV diagram).

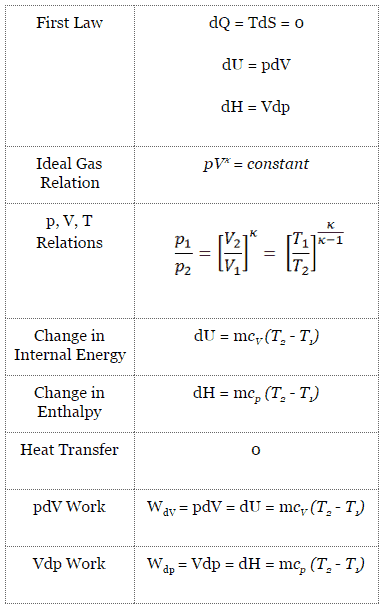
As can be seen, it is convenient to use enthalpy or specific enthalpy and to express the first law in terms of enthalpy in analysis of this thermodynamic cycle. This form of the law simplifies the description of energy transfer. At constant pressure, the enthalpy change equals the energy transferred from the environment through heating:

Isobaric process (Vdp = 0):

dH = dQ → Q = H₂ – H₁

At constant entropy, i.e. in isentropic process, the enthalpy change equals the flow process work done on or by the system:

Isentropic process (dQ = 0):

dH = Vdp → W = H₂ – H₁

Isentropic Process

An isentropic process is a thermodynamic process, in which the entropy of the fluid or gas remains constant. It means the isentropic process is a special case of an adiabatic process in which there is no transfer of heat or matter. It is a reversible adiabatic process. The assumption of no heat transfer is very important, since we can use the adiabatic approximation only in very rapid processes.

Isentropic Process and the First Law

For a closed system, we can write the first law of thermodynamics in terms of enthalpy:

dH = dQ + Vdp

dH = TdS + Vdp

Isentropic process (dQ = 0):

dH = Vdp → W = H₂ – H₁ → H₂ – H₁ = C_p (T₂ – T₁) (for ideal gas)

Isentropic Process of the Ideal Gas

The isentropic process (a special case of adiabatic process) can be expressed with the ideal gas law as:

pV^κ = constant

p₁V₁^κ = p₂V₂^κ

in which κ = c_p/c_v is the ratio of the specific heats (or heat capacities) for the gas. One for constant pressure (c_p) and one for constant volume (c_v). Note that, this ratio κ = c_p/c_v is a factor in determining the speed of sound in a gas and other adiabatic processes.

Isobaric Process

An isobaric process is a thermodynamic process, in which the pressure of the system remains constant (p = const). The heat transfer into or out of the system does work, but also changes the internal energy of the system.

Since there are changes in internal energy (dU) and changes in system volume (∆V), engineers often use the enthalpy of the system, which is defined as:

H = U + pV

Isobaric Process and the First Law

The classical form of the first law of thermodynamics is the following equation:

dU = dQ – dW

In this equation dW is equal to dW = pdV and is known as the boundary work. In an isobaric process and the ideal gas, part of heat added to the system will be used to do work and part of heat added will increase the internal energy (increase the temperature). Therefore it is convenient to use the enthalpy instead of the internal energy.

Isobaric process (Vdp = 0):

dH = dQ → Q = H₂– H₁

At constant entropy, i.e. in isentropic process, the enthalpy change equals the flow process work done on or by the system.

Isobaric Process of the Ideal Gas

The isobaric process can be expressed with the ideal gas law as: