What is Ideal Gas Law – Definition

Ideal Gas Model

The ideal gas model is used to predict the behavior of gases and is one of the most useful and commonly used substance models ever developed. I was found, that if we confine 1 mol samples of various gases in identical volume and hold the gases at the same temperature, then their measured pressures are almost the same. Moreover when we confine gases at lower densities the differences tend to disappear. It was found, such gases tend to obey the following relation, which is known as the ideal gas law:

pV = nRT

where:

p is the absolute pressure of the gas

n is the amount of substance

T is the absolute temperature

V is the volume

R  is the ideal, or universal, gas constant, equal to the product of the Boltzmann constant and the Avogadro constant. The power of the ideal gas law is in its simplicity. When any two of the thermodynamic variables, p, v, and T are given, the third can easily be found.

The ideal gas model is based on following assumptions:

1. The pressure, volume, and temperature of an ideal gas obey the ideal gas law.
2. The specific internal energy is only a function of the temperature: u = u(T)
3. The molar mass of an ideal gas is identical with the molar mass of the real substance
4. The specific heats — c_p and c_v — are independent of temperature which means that they are constants.

From microscopic point of view it is based on these assumptions:

1. The molecules of the gas are small, hard spheres.
2. The only forces between the gas molecules are those that determine the point-like collisions.
3. All collisions are elastic and all motion is frictionless.
4. The average distance between molecules is much larger than the size of the molecules.
5. The molecules are moving in random directions.
6. There are no other attractive or repulsive force between these molecules.

What is an Ideal Gas

An ideal gas is defined as one in which all collisions between atoms or molecules are perfectly elastic and in which there are no intermolecular attractive forces. An ideal gas can be visualized as a collection of perfectly hard spheres which collide but which otherwise do not interact with each other. In reality, no real gases are like an ideal gas and therefore no real gases follow the ideal gas law or equation completely. At temperatures near a gases boiling point, increases in pressure will cause condensation to take place and drastic decreases in volume. At very high pressures, the intermolecular forces of a gas are significant. However, most gases are in approximate agreement at pressures and temperatures above their boiling point. The ideal gas law is utilized by engineers working with gases because it is simple to use and approximates real gas behavior.

See also: Elastic Collision

Joule’s Second Law

For any gas whose equation of state is given exactly by pV = nRT (or pv = RT), the specific internal energy depends on temperature only. This rule was originally found in 1843 by an English physicist James Prescott Joule experimentally for real gases and is known as Joule’s second law:

The internal energy of a fixed mass of an ideal gas depends only on its temperature (not pressure or volume).

The specific enthalpy of a gas described by pV = nRT also depends on temperature only. Note that, the enthalpy is the thermodynamic quantity equivalent to the total heat content of a system. It is equal to the internal energy of the system plus the product of pressure and volume. In intensive variables the Joule’s second law is therefore given by h = h(T) = u(T) + pv = u(T) + RT.

These three equations constitute the ideal gas model, summarized as follows:

pv = RT

u = u(T)

h = h(T) = u(T) + RT

Ideal Gas Law

Any equation that relates the pressure, temperature, and specific volume of a substance is called an equation of state. The simplest and best-known equation of state for substances in the gas phase is the Ideal Gas equation of state. It was first stated by Émile Clapeyron in 1834 as a combination of the empirical Boyle’s law, Charles’ law and Avogadro’s Law. This equation predicts the p-v-T behavior of a gas quite accurately for dilute or low-pressure gases. In an ideal gas, molecules have no volume and do not interact. According to the ideal gas law, pressure varies linearly with temperature and quantity, and inversely with volume.

pV = nRT

where:

• p is the absolute pressure of the gas
• n is the amount of substance
• T is the absolute temperature
• V is the volume
• R  is the ideal, or universal, gas constant, equal to the product of the Boltzmann constant and the Avogadro constant,

In this equation the symbol R is a constant called the universal gas constant that has the same value for all gases—namely, R =  8.31 J/mol K.

The power of the ideal gas law is in its simplicity. When any two of the thermodynamic variables, p, v, and T are given, the third can easily be found. Many physical conditions of gases calculated by engineers fit the above description. Perhaps the most common use of gas behavior studied by engineers is that of the compression process and expansion process using ideal gas approximations.

Gas Laws

In general, the gas laws are first equations of state, that correlate densities of gases and liquids to temperatures and pressures. The gas laws were completely developed at the end of the 18th century. These laws or statements preceded the ideal gas law, since individually these laws are considered as special cases of the ideal gas equation, with one or more of the variables held constant. Since they have been almost completely replaced by the ideal gas equation, it is not usual for students to learn these laws in detail. The ideal gas equation was first stated by Émile Clapeyron in 1834 as a combination of these laws:

• Boyle-Mariotte Law
• Charles’s Law
• Guy-Lussac’s Law
• Avogadro’s Law

Example: Ideal Gas Law – Gas compression inside a pressurizer

Pressure in the primary circuit of PWRs is maintained by a pressurizer, a separate vessel that is connected to the primary circuit (hot leg) and partially filled with water which is heated to the saturation temperature (boiling point) for the desired pressure by submerged electrical heaters. During the plant heatup the pressurizer can be filled by nitrogen instead of saturated steam.

Assume that a pressurizer contains 12 m³ of nitrogen at 20°C and 15 bar. The temperature is raised to 35°C, and the volume is reduced to 8.5 m³. What is the final pressure of the gas inside the pressurizer? Assume that the gas is ideal.

Solution:

Since the gas is ideal,we can use the ideal gas law to relate its parameters, both in the initial state i and in the final state f. Therefore:

p_initV_init = nRT_init

and

p_finalV_final = nRT_final

Dividing the second equation by the first equation and solving for p_f we obtain:

p_final = p_initT_finalV_init / T_initV_final

Note that we cannot convert units of volume and pressure to basic SI units, because they cancel out each other. On the other hand we have to use Kelvins instead of degrees of Celsius. Therefore T_init = 293 K and T_final = 308 K.

It follows, the resulting pressure in the final state will be:

p_final = (15 bar) x (308 K) x (12 m³) / (293 K) x (8.5 m³) = 22 bar

Validity of Ideal Gas Law

Since ideal gas is defined as one in which all collisions between atoms or molecules are perfectly elastic and in which there are no intermolecular attractive forces, there is no such thing in nature as a truly ideal gas. On the other hand, all real gases approach the ideal state at low pressures (densities). At low pressures molecules are far enough apart that they do not interact with one another.

In other words, the Ideal Gas Law is accurate only at relatively low pressures (relative to the critical pressure p_cr) and high temperatures (relative to the critical temperature T_cr). At these parameters, the compressibility factor, Z = pv / RT, is approximately 1. The compressibility factor is used to account for deviation from the ideal situation. This correction factor is dependent on pressure and temperature for each gas considered.

Internal Energy of an Ideal Gas

The internal energy is the total of all the energy associated with the motion of the atoms or molecules in the system. Microscopic forms of energy include those due to the rotation, vibration, translation, and interactions among the molecules of a substance.

Monatomic Gas – Internal Energy

For a monatomic ideal gas (such as helium, neon, or argon), the only contribution to the energy comes from translational kinetic energy. The average translational kinetic energy of a single atom depends only on the gas temperature and is given by equation:

K_avg = 3/2 kT.

The internal energy of n moles of an ideal monatomic (one atom per molecule) gas is equal to the average kinetic energy per molecule times the total number of molecules, N:

E_int = 3/2 NkT = 3/2 nRT

where n is the number of moles. Each direction (x, y, and z) contributes (1/2)nRT to the internal energy. This is where the equipartition of energy idea comes in – any other contribution to the energy must also contribute (1/2)nRT. As can be seen, the internal energy of an ideal gas depends only on temperature and the number of moles of gas.

Diatomic Molecule – Internal Energy

If the gas molecules contain more than one atom, there are three translation directions, and rotational kinetic energy also contributes, but only for rotations about two of the three perpendicular axes. The five contributions to the energy (five degrees of freedom) give:

Diatomic ideal gas:

E_int = 5/2 NkT = 5/2 nRT

This is only an approximation and applies at intermediate temperatures. At low temperatures only the translational kinetic energy contributes, and at higher temperatures two additional contributions (kinetic and potential energy) come from vibration. The internal energy will be greater at a given temperature than for a monatomic gas, but it will still be a function only of temperature for an ideal gas.

The internal energy of real gases also depends mainly on temperature, but similarly as the Ideal Gas Law, the internal energy of real gases depends also somewhat on pressure and volume. All real gases approach the ideal state at low pressures (densities). At low pressures molecules are far enough apart that they do not interact with one another. The internal energy of liquids and solids is quite complicated, for it includes electrical potential energy associated with the forces (or chemical bonds) between atoms and molecules.

Specific Heat at Constant Volume and Constant Pressure

Specific heat is a property related to internal energy that is very important in thermodynamics. The intensive properties c_v and c_p are defined for pure, simple compressible substances as partial derivatives of the internal energy u(T, v) and enthalpy h(T, p), respectively:

where the subscripts v and p denote the variables held fixed during differentiation. The properties c_v and c_p are referred to as specific heats (or heat capacities) because under certain special conditions they relate the temperature change of a system to the amount of energy added by heat transfer. Their SI units are J/kg K or J/mol K. Two specific heats are defined for gases, one for constant volume (c_v) and one for constant pressure (c_p).

According to the first law of thermodynamics, for constant volume process with a monatomic ideal gas the molar specific heat will be:

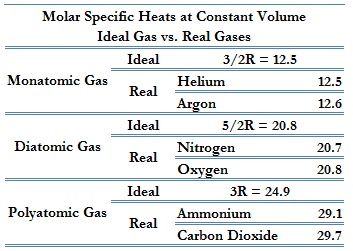
C_v = 3/2R = 12.5 J/mol K

because

U = 3/2nRT

It can be derived that the molar specific heat at constant pressure is:

C_p = C_v + R = 5/2R = 20.8 J/mol K

This C_p is greater than the molar specific heat at constant volume C_v, because energy must now be supplied not only to raise the temperature of the gas but also for the gas to do work because in this case volume changes.

Mayer’s relation – Mayer’s formula

Julius Robert Mayer, a German chemist and physicist, derived a relation between specific heat at constant pressure and the specific heat at constant volume for an ideal gas. He studied the fact that the specific heat capacity of a gas at constant pressure (C_p) is slightly greater than at constant volume (C_v). He reasoned that this C_p is greater than the molar specific heat at constant volume C_v, because energy must now be supplied not only to raise the temperature of the gas but also for the gas to do work because in this case volume changes. According to the Mayer’s relation or the Mayer’s formula the difference between these two heat capacities is equal to the universal gas constant, thus the molar specific heat at constant pressure is equal:

C_p = C_v + R

See also:

Thermodynamics