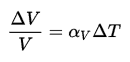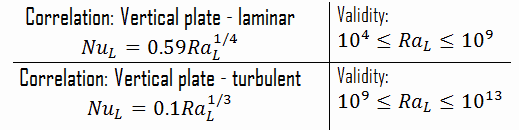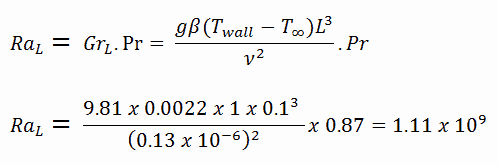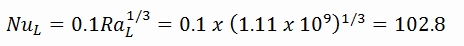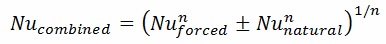Natural Convection – Heat Transfer
Similarly as for forced convection, also natural convection heat transfer take place both by thermal diffusion (the random motion of fluid molecules) and by advection, in which matter or heat is transported by the larger-scale motion of currents in the fluid. At the surface, energy flow occurs purely by conduction, even in convection. It is due to the fact, there is always a thin stagnant fluid film layer on the heat transfer surface. But in the next layers both conduction and diffusion-mass movement in the molecular level or macroscopic level occurs. Due to the mass movement the rate of energy transfer is higher. Higher the rate of mass movement, thinner the stagnant fluid film layer will be and higher will be the heat flow rate.
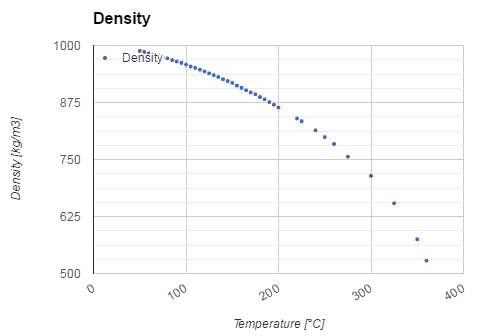
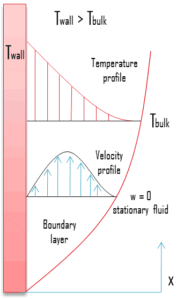 We know that the density of gases and liquids depends on temperature, generally decreasing (due to fluid expansion) with increasing temperature.
We know that the density of gases and liquids depends on temperature, generally decreasing (due to fluid expansion) with increasing temperature.
The magnitude of the natural convection heat transfer between a surface and a fluid is directly related to the flow rate of the fluid induced by natural convection. The higher the flow rate, the higher the heat transfer rate. The flow rate in case of natural convection is established by the dynamic balance of buoyancy and friction.
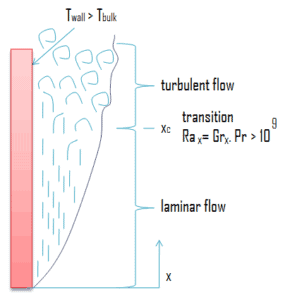
Assume a plate at the temperature Twall, which is immersed in a quiescent fluid at the temperature Tbulk, where (Twall > Tbulk). The fluid close to the plate is less dense than fluid that is further removed. Buoyancy forces therefore induce a natural convection boundary layer in which the heated and lighter fluid rises vertically, entraining heavier fluid from the quiescent region. The resulting velocity distribution is unlike that associated with forced convection boundary layers and depends also on the fluid viscosity. In particular, the velocity is zero at the surface as well as at the boundary due to viscous forces. It must be noted, a natural convection also develops if (Twall < Tbulk), but, in this case, fluid motion will be downward.
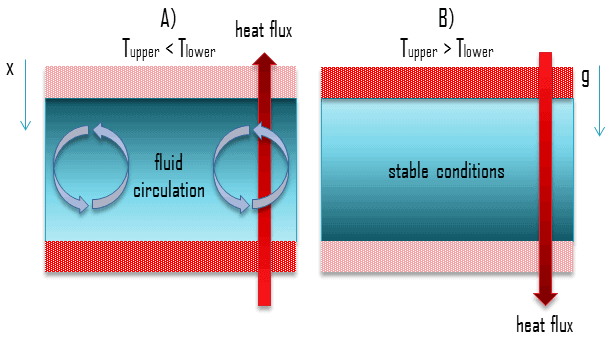
The presence and magnitude of natural convection also depend on the geometry of the problem. The presence of a fluid density gradient in a gravitational field does not ensure the existence of natural convection currents. This problem is illustrated in the following figure, where a fluid is enclosed by two large, horizontal plates of different temperature (Tupper ≠ Tlower).
- In case A the temperature of the lower plate is higher than the temperature of the upper plate. In this case, the density decreases in the direction of the gravitational force. This geometry induces fluid circulation and heat transfer occurs via natural circulation. The heavier fluid will descend, being warmed in the process, while the lighter fluid will rise, cooling as it moves.
- In case B the temperature of the lower plate is lower than the temperature of the upper plate. In this case, the density increases in the direction of the gravitational force. This geometry leads to stable conditions, stable temperature gradient and does not induce fluid circulation. Heat transfer occurs solely via thermal conduction.
Since the natural convection is strongly dependent on the geometry, most heat transfer correlations in natural convection are based on experimental measurements and engineers often use proper characteristic numbers to describe natural convection heat transfer.
Natural Convection – Correlations
As was written, most heat transfer correlations in natural convection are based on experimental measurements and engineers often use proper characteristic numbers to describe natural convection heat transfer. The characteristic number that describes convective heat transfer (i.e. the heat transfer coefficient) is the Nusselt number, which is defined as the ratio of the thermal energy convected to the fluid to the thermal energy conducted within the fluid. The Nusselt number represents the enhancement of heat transfer through a fluid layer as a result of convection relative to conduction across the same fluid layer. But in case of free convection, heat transfer correlations (for the Nusselt number) are usually expressed in terms of the Rayleigh number.
The Rayleigh number is used to express heat transfer in natural convection. The magnitude of the Rayleigh number is a good indication as to whether the natural convection boundary layer is laminar or turbulent. The simple empirical correlations for the average Nusselt number, Nu, in natural convection are of the form:
Nux = C. Raxn
The values of the constants C and n depend on the geometry of the surface and the flow regime, which is characterized by the range of the Rayleigh number. The value of n is usually n = 1/4 for laminar flow and n = 1/3 for turbulent flow.
For example:
See also: Nusselt Number
See also: Rayleigh Number
Example: Natural Convection – Flat Plate
A 10cm high vertical plate is maintained at 261°C in 260°C compressed water (16MPa). Determine the Nusselt number using the simple correlation for a vertical flat plate.
To calculate the Rayleigh number, we have to know:
- the coefficient of thermal expansion, which is: β = 0.0022
- the Prandtl number (for 260°C), which is: Pr = 0.87
- the kinematic viscosity (for 260°C), which is ν = 0.13 x 10-6 (note that, this value is significantly lower than that for 20°C)
The resulting Rayleigh number is:
The resulting Nusselt number, which represents the enhancement of heat transfer through a fluid layer as a result of convection relative to conduction across the same fluid layer is:
Combined Forced and Natural Convection
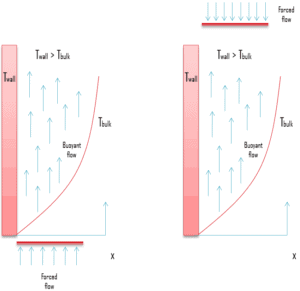 As was written, convection takes place through advection, diffusion or both. In preceding chapters we considered convection transfer in fluid flows that originate from an external forcing condition – forced convection. In this chapter, we consider natural convection, where any fluid motion occurs by natural means such as buoyancy. In fact, there are flow regimes, in which we have to consider both forcing mechanisms. When flow velocities are low, natural convection will also contribute in addition to forced convection. Whether or not free convection is significant for heat transfer, it can be checked using the following criteria:
As was written, convection takes place through advection, diffusion or both. In preceding chapters we considered convection transfer in fluid flows that originate from an external forcing condition – forced convection. In this chapter, we consider natural convection, where any fluid motion occurs by natural means such as buoyancy. In fact, there are flow regimes, in which we have to consider both forcing mechanisms. When flow velocities are low, natural convection will also contribute in addition to forced convection. Whether or not free convection is significant for heat transfer, it can be checked using the following criteria:
- If Gr/Re2 >> 1 free convection prevails
- If Gr/Re2 << 1 forced convection prevails
- If Gr/Re2 ≈ 1 both should be considered
The effect of buoyancy on heat transfer in a forced flow is strongly influenced by the direction of the buoyancy force relative to that of the flow. Natural convection may help or hurt forced convection heat transfer, depending on the relative directions of buoyancy-induced and the forced convection motions. Three special cases that have been studied extensively correspond to buoyancy-induced and forced motions:
- Assisting flow. The buoyant motion is in the same direction as the forced motion.
- Opposing flow. The buoyant motion is in the opposite direction to the forced motion.
- Transverse flow. The buoyant motion is perpendicular to the forced motion.
It is obvious, in assisting and transverse flows, buoyancy enhances the rate of heat transfer associated with pure forced convection. On the other hand, in opposing flows, it decreases the rate of heat transfer. When determining the Nusselt number under combined natural and forced convection conditions, it is tempting to add the contributions of natural and forced convection in assisting flows and to subtract them in opposing flows:
For the specific geometry of interest, the Nusselt numbers Nuforced and Nunatural are determined from existing correlations for pure forced and natural (free) convection, respectively. The best correlation of data to experiments is often obtained for exponent n = 3, but it may vary between 3 and 4, depending on the geometry of the problem.
We hope, this article, Natural Convection – Heat Transfer – Correlations, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.