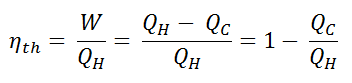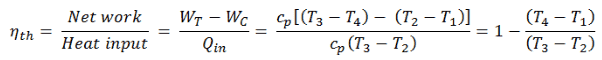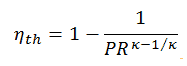Thermal Efficiency of Brayton Cycle
In general the thermal efficiency, ηth, of any heat engine is defined as the ratio of the work it does, W, to the heat input at the high temperature, QH.
The thermal efficiency, ηth, represents the fraction of heat, QH, that is converted to work. Since energy is conserved according to the first law of thermodynamics and energy cannot be be converted to work completely, the heat input, QH, must equal the work done, W, plus the heat that must be dissipated as waste heat QC into the environment. Therefore we can rewrite the formula for thermal efficiency as:
This is very useful formula, but here we express the thermal efficiency using the first law in terms of enthalpy.
To calculate the thermal efficiency of the Brayton cycle (single compressor and single turbine) engineers use the first law of thermodynamics in terms of enthalpy rather than in terms of internal energy.
The first law in terms of enthalpy is:
dH = dQ + Vdp
In this equation the term Vdp is a flow process work. This work, Vdp, is used for open flow systems like a turbine or a pump in which there is a “dp”, i.e. change in pressure. There are no changes in control volume. As can be seen, this form of the law simplifies the description of energy transfer.
There are expressions in terms of more familiar variables such as temperature and pressure:
dH = CpdT + V(1-αT)dp
Where Cp is the heat capacity at constant pressure and α is the coefficient of (cubic) thermal expansion. For ideal gas αT = 1 and therefore:
dH = CpdT
At constant pressure, the enthalpy change equals the energy transferred from the environment through heating:
Isobaric process (Vdp = 0):
dH = dQ → Q = H3 – H2 → H3 – H2 = Cp (T3 – T2)
At constant entropy, i.e. in isentropic process, the enthalpy change equals the flow process work done on or by the system:
Isentropic process (dQ = 0):
dH = Vdp → W = H4 – H3 → H4 – H3 = Cp (T4 – T3)
The enthalpy can be made into an intensive, or specific, variable by dividing by the mass. Engineers use the specific enthalpy in thermodynamic analysis more than the enthalpy itself.
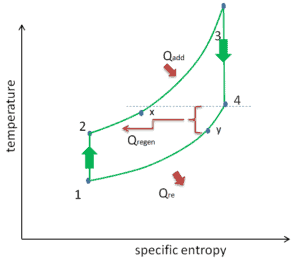
Now, let assume the ideal Brayton cycle that describes the workings of a constant pressure heat engine. Modern gas turbine engines and airbreathing jet engines also follow the Brayton cycle. This cycle consist of four thermodynamic processes:
-
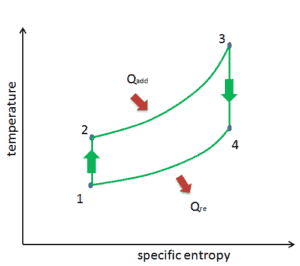
Brayton Cycle – Ts diagram isentropic compression – ambient air is drawn into the compressor, where it is pressurized (1 → 2). The work required for the compressor is given by WC = H2 – H1.
- isobaric heat addition – the compressed air then runs through a combustion chamber, where fuel is burned and air or another medium is heated (2 → 3). It is a constant-pressure process, since the chamber is open to flow in and out. The net heat added is given by Qadd = H3 – H2
- isentropic expansion – the heated, pressurized air then expands on turbine, gives up its energy. The work done by turbine is given by WT = H4 – H3
- isobaric heat rejection – the residual heat must be rejected in order to close the cycle. The net heat rejected is given by Qre = H4 – H1
As can be seen, we can fully describe and calculate such cycles (similarly for Rankine cycle) using enthalpies.
Thermal Efficiency – Brayton Cycle
The thermal efficiency of such simple Brayton cycle, for ideal gas can now be expressed in terms of the temperatures:
where
- WT the work done by the gas in the turbine
- WC the work done on the gas in the compressor
- cp is the heat capacity ratio
Pressure Ratio – Brayton Cycle – Gas Turbine
The thermal efficiency in terms of the compressor pressure ratio (PR = p2/p1), which is the parameter commonly used:
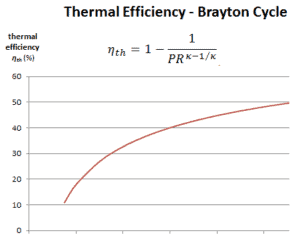In general, increasing the pressure ratio is the most direct way to increase the overall thermal efficiency of a Brayton cycle, because the cycle approaches the Carnot cycle.
According to Carnot’s principle higher efficiencies can be attained by increasing the temperature of the gas.
But there are also limits on the pressure ratios that can be used in the cycle. The highest temperature in the cycle occurs at the end of the combustion process, and it is limited by the maximum temperature that the turbine blades can withstand. As usual, metallurgical considerations (about 1700 K) place an upper limits on thermal efficiency.
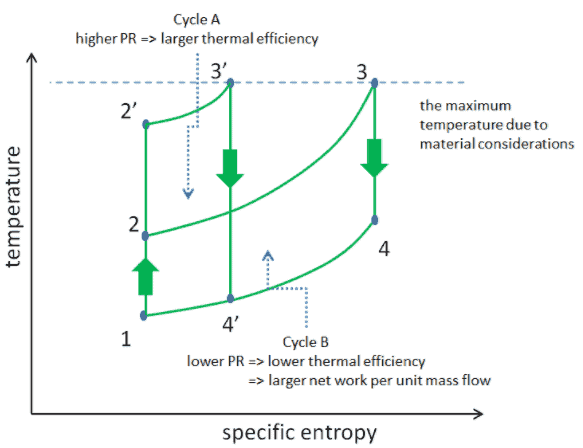
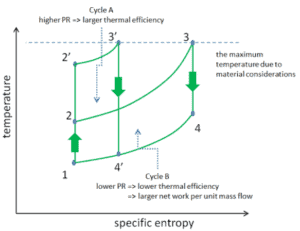
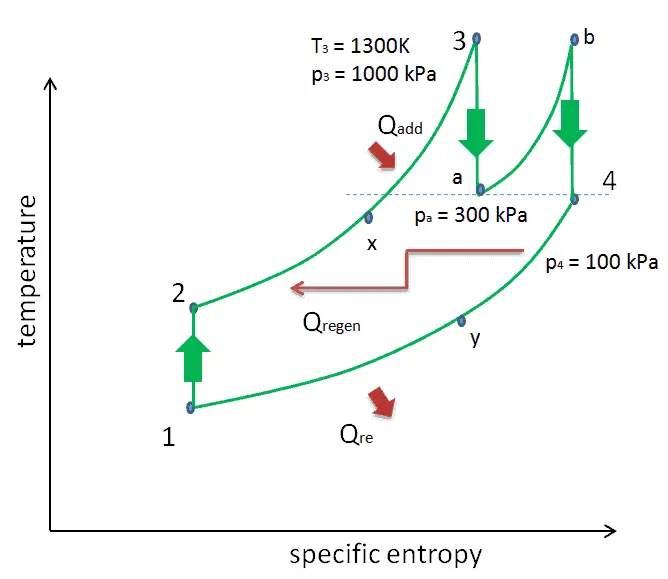
Consider the effect of compressor pressure ratio on thermal efficiency when the turbine inlet temperature is restricted to the maximum allowable temperature. There are two Ts diagrams of Brayton cycles having the same turbine inlet temperature but different compressor pressure ratios on the picture. As can be seen for a fixed-turbine inlet temperature, the net work output per cycle (Wnet = WT – WC) decreases with the pressure ratio (Cycle A). But the Cycle A has the greater efficiency.
On the other hand, the Cycle B has a larger net work output per cycle (enclosed area in the diagram) and thus the greater net work developed per unit of mass flow. The work produced by the cycle times a mass flow rate through the cycle is equal to the power output produced by the gas turbine.
Therefore with less work output per cycle (Cycle A), a larger mass flow rate (thus a larger system) is needed to maintain the same power output, which may not be economical. This is the key consideration in design of gas turbine, since here engineers must balance the thermal efficiency and the compactness. In most common designs, the pressure ratio of a gas turbine ranges from about 11 to 16.
Thermal Efficiency Improvement – Brayton Cycle
There are several methods, how can be the thermal efficiency of the Brayton cycle improved. Assuming that the maximum temperature is limited by metallurgical consideration, these methods are:
As was discussed, increasing the pressure ratio increases the compressor discharge temperature. Since the turbine inlet temperature is limited by the maximum temperature that the turbine blades can withstand, the pressure ration influences the heat amount that can be added to the flow. Moreover with an increase of the pressure ratio, the diameter of the compressor blades becomes progressively smaller in higher pressure stages of the compressor. Because the gap between the blades and the engine casing increases in size as a percentage of the compressor blade height as the blades get smaller in diameter, a greater percentage of the compressed air can leak back past the blades in higher pressure stages. This causes a leak back and in result it decreases the isentropic compressor efficiency (will be discussed later). Finally, from the formula for the thermal efficiency in term of pressure ratio can be seen, there is smaller gain as the pressure ratio increases (due to the exponent).
Significant increases in the thermal efficiency of gas turbine power plants can be achieved through reducing the amount of fuel that must be burned in the combustion chamber. This can be done by transferring heat from the turbine exhaust gas, which is normally well above the ambient temperature, to the compressor discharge air flow known as heat regeneration. Especially at a low or moderate pressure ratio, there is a high temperature increase in the combustion chamber and the turbine exhaust gas might still contain significant amount of heat at higher temperature than the compressor outlet gas (after the last compression stage but before the combustor). For this purpose a heat exchanger called a regenerator is used. Sometimes engineers use the term economiser that are heat exchangers intended to reduce energy consumption, especially in case of preheating of a fluid.
This heat regenerator allows the air exiting the compressor to be preheated before it enters the combustion chamber, thereby reducing the amount of fuel that must be burned in the combustor. This form of heat recycling is only possible if the gas turbine is run with low pressure ratio.
As was stated the temperature difference between turbine outlet and compressor outlet is crucial and determines the amount of heat that can be recovered. In case of negative difference (i.e. T2 > T4), the heat regeneration is not possible. There are two main ways, how to change this difference:
- to increase the turbine outlet temperature (T4) through reheat of the flow during expansion phase (i.e. use of a multistage turbine with a reheat combustor or with a reheater)
- to decrease the compressor outlet temperature (T2) through intercooling of the flow during compression phase (i.e. use of a multistage compressor with an intercooler)
Therefore reheat and intercooling are complementary with heat regeneration. By itself, they would not necessarily increase the thermal efficiency, however, when intercooling or reheat is used in conjunction with heat regeneration, a significant increase in thermal efficiency can be achieved.
It must be noted, transferring heat from the turbine outlet to the compressor inlet would reduce efficiency, as hotter inlet air means more volume, thus more work for the compressor. Engineers must also take into consideration pressure losses generated by the heat exchanger that slightly reduce the power of the gas turbine.
Regeneration vs. Recuperation of Heat
In general, the heat exchangers used in regeneration may be classified as either regenerators or recuperators.
- Regenerator is a type of heat exchanger where heat from the hot fluid is intermittently stored in a thermal storage medium before it is transferred to the cold fluid. It has a single flow path in which the hot and cold fluids alternately pass through.
- Recuperator is a type of heat exchanger has separate flow paths for each fluid along their own passages and heat is transferred through the separating walls. Recuperators (e.g. economisers) are often used in power engineering, to increase the overall efficiency of thermodynamic cycles. For example, in a gas turbine engine. The recuperator transfers some of the waste heat in the exhaust to the compressed air, thus preheating it before entering the combustion chamber. Many recuperators are designed as counterflow heat exchangers.
As was discussed, the maximum temperature is limited by metallurgical consideration, but in order to deliver more of the heat at a temperature close to the peak of the cycle the gas can be reheated in a reheater. This involves splitting the turbine, i.e. use of a multistage turbine with a reheat combustor or with a reheater. High pressure and low pressure stages of the turbine may be on the same shaft to drive a common generator, but they will have separate cases. With a reheater, the flow is extracted after a partial expansion (point a), run back through the heat exchanger to heat it back up to the peak temperature (point b), and then passed to the lower pressure stage of the turbine. The expansion then completed in this stage from point b to point 4.
With this arrangement the net work per unit of mass flow can be increased. Despite the increase in net work with reheat, the cycle thermal efficiency would not necessarily increase because a greater total heat addition would be required. On the other hand, the temperature at the exit of the turbine (low pressure stage) is higher with reheat than without reheat, so there is the potential for heat regeneration. Therefore reheat and regeneration are complementary, they are used usually together in order to increase the thermal efficiency of gas turbine.
Significant increases in the thermal efficiency of gas turbine power plants can be achieved also through intercooling. Intercooling can be applied between compressor stages to reduce compression work, WC, hence increasing overall power of the gas turbine.
For this purpose a heat exchanger known as an intercooler is usually used between stages of a multi-stage compression process. In general, intercoolers are heat exchangers that are used in many applications, including air compressors, air conditioners, refrigerators, and gas turbines. Intercoolers are widely known also in automotive use as a turbocharger or supercharger, but here they increase intake air charge density, hence the power of an engine.
In gas turbine power plant the thermal efficiency is of the highest importance and intercooling with heat regeneration are widely used. This involves splitting the compressor, i.e. use of a multistage compressor with an intercooler or intercoolers. High pressure and low pressure stages of the compressor may be on the same shaft even with turbine or a generator, but it is not a rule. With an intercooler, the flow is extracted after a partial compression (point c), run through the heat exchanger (intercooler) to cool it to the ambient temperature (point d), and then passed to the high stage of compressor. The compression is then completed in the second compressor from point d to point 2.
With this arrangement the net work per unit of mass flow (↑Wnet = WT – ↓WC) can be increased by reducing the compression work (↓WC). Despite the increase in net work with intercooling, the cycle thermal efficiency would not necessarily increase because the temperature of the air entering the combustor would be reduced and a greater total heat addition would be required to achieve the desired turbine inlet temperature. On the other hand, the temperature at the exit of the compressor (high pressure stage) is lower with intercooling than without intercooling, so there is the potential for heat regeneration (Qregen increases). Note that, the heat regeneration requires lower compressor outlet temperature than the turbine outlet temperature (simply due to 2nd law) and this temperature difference determines the amount of heat available for heat regeneration.
Therefore reheat and intercooling are complementary with heat regeneration. By itself, they would not necessarily increase the thermal efficiency, however, when intercooling or reheat is used in conjunction with heat regeneration, a significant increase in thermal efficiency can be achieved.
Some large compressors with higher pressure ratio have several stages of compression with intercooling between stages. Engineers must also take into consideration pressure losses generated by all heat exchangers that slightly increase compression work. The certain gas turbine design (the number of intercoolers, reheaters and regenerators) is an engineering problem and depends on certain purpose of the gas turbine.
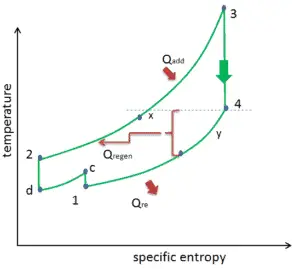
Reheat, Intercooling and Regeneration in Brayton Cycle
As was discussed reheat and intercooling are complementary with heat regeneration. By itself, they would not necessarily increase the thermal efficiency, however, when intercooling or reheat is used in conjunction with heat regeneration, a significant increase in thermal efficiency can be achieved and the net work output is also increased. This requires a gas turbine with two stages of compression and two turbine stages.
The second Ericsson cycle is similar to the Brayton cycle, but uses external heat and incorporates the multiple use of an intercooling and reheat. In fact, it is like a Brayton cycle with an infinite number of reheat and intercooler stages in the cycle. Compared to the Brayton cycle which uses adiabatic compression and expansion, an ideal Ericsson cycle consists of isothermal compression and expansion processes, combined with isobaric heat regeneration between them. Applying intercooling, heat regeneration and sequential combustion significantly increases thermal efficiency of a turbine, in fact, the thermal efficiency of the ideal Ericsson cycle equals to the Carnot efficiency.
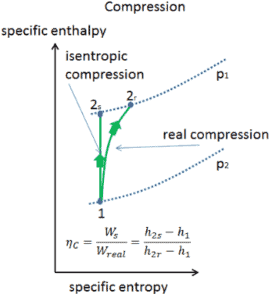
Isentropic Efficiency – Turbine, Compressor
Most steady-flow devices (turbines, compressors, nozzles) operate under adiabatic conditions, but they are not truly isentropic but are rather idealized as isentropic for calculation purposes. We define parameters ηT, ηC, ηN, as a ratio of real work done by device to work by device when operated under isentropic conditions (in case of turbine). This ratio is known as the Isentropic Turbine/Compressor/Nozzle Efficiency.
See also: Irreversibility of Natural Processes
These parameters describe how efficiently a turbine, compressor or nozzle approximates a corresponding isentropic device. This parameter reduces the overall efficiency and work output. For turbines, the value of ηT is typically 0.7 to 0.9 (70–90%).
Assume an isentropic expansion of helium (3 → 4) in a gas turbine. In this turbines the high-pressure stage receives gas (point 3 at the figure; p3 = 6.7 MPa; T3 = 1190 K (917°C)) from a heat exchanger and exhaust it to another heat exchanger, where the outlet pressure is p4 = 2.78 MPa (point 4). The temperature (for isentropic process) of the gas at the exit of the turbine is T4s = 839 K (566°C).
Calculate the work done by this turbine and calculate the real temperature at the exit of the turbine, when the isentropic turbine efficiency is ηT = 0.91 (91%).
Solution:
From the first law of thermodynamics, the work done by turbine in an isentropic process can be calculated from:
WT = h3 – h4s → WTs = cp (T3 – T4s)
From Ideal Gas Law we know, that the molar specific heat of a monatomic ideal gas is:
Cv = 3/2R = 12.5 J/mol K and Cp = Cv + R = 5/2R = 20.8 J/mol K
We transfer the specific heat capacities into units of J/kg K via:
cp = Cp . 1/M (molar weight of helium) = 20.8 x 4.10-3 = 5200 J/kg K
The work done by gas turbine in isentropic process is then:
WT,s = cp (T3 – T4s) = 5200 x (1190 – 839) = 1.825 MJ/kg
The real work done by gas turbine in adiabatic process is then:
WT,real = cp (T3 – T4s) . ηT = 5200 x (1190 – 839) x 0.91 = 1.661 MJ/kg
We hope, this article, Thermal Efficiency – Brayton Cycle, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.