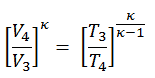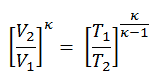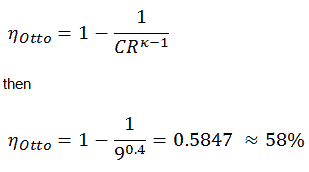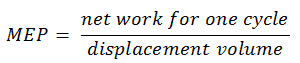Facebook Instagram Youtube Twitter

What is Otto Cycle – Problem with Solution – Definition

by
Otto Cycle – Problem with Solution – Example. Let assume the Otto cycle. Calculate key characteristics such as mass of intake air, pressures and MEP. Thermal Engineering

Otto Cycle – Problem with Solution

Let assume the Otto cycle, which is the one of most common thermodynamic cycles that can be found in automobile engines. One of key parameters of such engines is the change in volumes between top dead center (TDC) to bottom dead center (BDC). The ratio of these volumes (V1 / V2) is known as the compression ratio.

The compression ratio in a gasoline-powered engine will usually not be much higher than 10:1 due to potential engine knocking (autoignition) and not lower than 6:1. For example, some sportscar engines can have compression ratio up to 12.5 : 1 (e.g. Ferrari 458 Italia).

Otto Cycle - PV Diagram
pV diagram of Otto Cycle. The area bounded by the complete cycle path represents the total work that can be done during one cycle.

In this example let assume an Otto cycle with compression ratio of CR = 9 : 1. The intake air is at 100 kPa = 1 bar, 20 °C, and the volume of the chamber is 500 cm³ prior to the compression stroke. The temperature at the end of adiabatic expansion is T4 = 800 K.

  • Specific heat capacity at constant pressure of air at atmospheric pressure and room temperature: cp = 1.01 kJ/kgK.
  • Specific heat capacity  at constant volume of air at atmospheric pressure and room temperature: cv = 0.718 kJ/kgK.
  • κ = cp/cv = 1.4

Calculate:

  1. the mass of intake air
  2. the temperature T3
  3. the pressure p3
  4. the amount of heat added by burning of fuel-air mixture
  5. the thermal efficiency of this cycle
  6. the MEP

Solution:

1) the mass of intake air

At the beginning of calculations we have to determine the amount of gas in the cylinder before the compression stroke. Using the ideal gas law, we can find the mass:

pV = mRspecificT

where:

  • p is the absolute pressure of the gas
  • m is the mass of substance
  • T is the absolute temperature
  • V is the volume
  • Rspecific  is the specific gas constant, equal to the universal gas constant divided by the molar mass (M) of the gas or mixture. For dry air Rspecific = 287.1 J.kg-1.K-1.

therefore

m = p1V1/RspecificT1 = (100000 × 500×10-6 )/(287.1 × 293) = 5.95×10-4 kg

In this problem all volumes are known:

  • V1 = V4 = Vmax = 500×10-6 m3 (0.5l)
  • V2 = V3 = Vmin = Vmax / CR = 55.56 ×10-6 m3

Note that (Vmax – Vmin) x number of cylinders = total engine displacement.

2) the temperature T3

Since the process is adiabatic, we can use the following p, V, T relation for adiabatic processes:

adiabatic formula - example

thus

T3 = T4 . CRκ – 1 = 800 . 90.4 = 1926 K

3) the pressure p3

Again, we can use the ideal gas law to find the pressure at the beginning of the power stroke as:

p3 = mRspecificT3 / V3 = 5.95×10-4 x 287.1 x 1926 / 55.56 ×10-6 = 5920000 Pa = 59.2 bar

4) the amount of heat added

To calculate the amount of heat added by burning of fuel-air mixture, Qadd, we have to use the first law of thermodynamics for isochoric process, which states the Qadd = ∆U, therefore:

Qadd = mcv (T3 – T2)

the temperature at the end of the compression stroke can be determined using the p, V, T relation for adiabatic processes between points 1 → 2.

adiabatic process - formula

T2 = T1 . CRκ – 1 = 293 . 90.4 = 706 K

then

Qadd = mcv (T3 – T2) = 5.95×10-4 x 718 x 1220 = 521.2 J

5) the thermal efficiency

Thermal efficiency for an Otto cycle:

As was derived in the previous section, the thermal efficiency of an Otto cycle is a function of compression ratio and κ:

Otto cycle - efficiency - example

6) the mean effective pressure

The MEP was defined as:

mean effective pressure - definition

It this equation the displacement volume is equal to Vmax – Vmin. The net work for one cycle can be calculated using the heat added and the thermal efficiency:

Wnet = Qadd . ηOtto = 521.2 x 0.5847 = 304.7 J
MEP = 304.7 / (500×10-6 – 55.56 ×10-6) = 685.6 kPa = 6.856 bar

 
References:
Nuclear and Reactor Physics:
  1. J. R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).
  2. J. R. Lamarsh, A. J. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 0-201-82498-1.
  3. W. M. Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1.
  4. Glasstone, Sesonske. Nuclear Reactor Engineering: Reactor Systems Engineering, Springer; 4th edition, 1994, ISBN: 978-0412985317
  5. W.S.C. Williams. Nuclear and Particle Physics. Clarendon Press; 1 edition, 1991, ISBN: 978-0198520467
  6. Kenneth S. Krane. Introductory Nuclear Physics, 3rd Edition, Wiley, 1987, ISBN: 978-0471805533
  7. G.R.Keepin. Physics of Nuclear Kinetics. Addison-Wesley Pub. Co; 1st edition, 1965
  8. Robert Reed Burn, Introduction to Nuclear Reactor Operation, 1988.
  9. U.S. Department of Energy, Nuclear Physics and Reactor Theory. DOE Fundamentals Handbook, Volume 1 and 2. January 1993.

Advanced Reactor Physics:

  1. K. O. Ott, W. A. Bezella, Introductory Nuclear Reactor Statics, American Nuclear Society, Revised edition (1989), 1989, ISBN: 0-894-48033-2.
  2. K. O. Ott, R. J. Neuhold, Introductory Nuclear Reactor Dynamics, American Nuclear Society, 1985, ISBN: 0-894-48029-4.
  3. D. L. Hetrick, Dynamics of Nuclear Reactors, American Nuclear Society, 1993, ISBN: 0-894-48453-2.
  4. E. E. Lewis, W. F. Miller, Computational Methods of Neutron Transport, American Nuclear Society, 1993, ISBN: 0-894-48452-4.

See also:

Otto Cycle

We hope, this article, Otto Cycle – Problem with Solution, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.

Related Posts