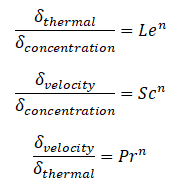Prandtl Number
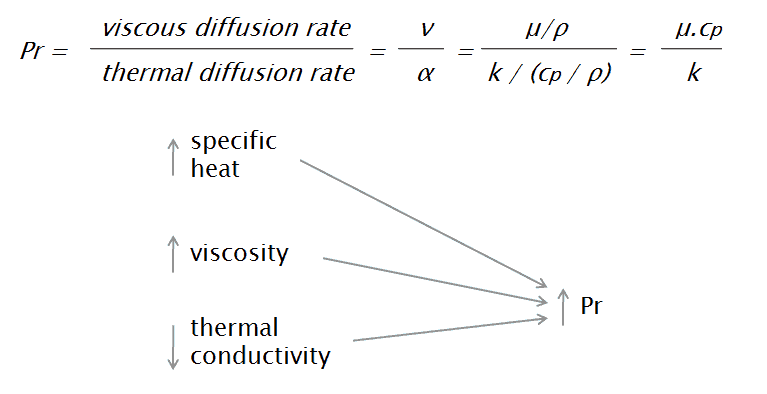
The Prandtl number is a dimensionless number, named after its inventor, a German engineer Ludwig Prandtl, who also identified the boundary layer. The Prandtl number is defined as the ratio of momentum diffusivity to thermal diffusivity. The momentum diffusivity, or as it is normally called, kinematic viscosity, tells us the material’s resistance to shear-flows (different layers of the flow travel with different velocities due to e.g. different speeds of adjacent walls) in relation to density. That is, the Prandtl number is given as:
where:
ν is momentum diffusivity (kinematic viscosity) [m2/s]
α is thermal diffusivity [m2/s]
μ is dynamic viscosity [N.s/m2]
k is thermal conductivity [W/m.K]
cp is specific heat [J/kg.K]
ρ is density [kg/m3]
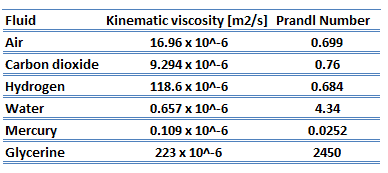
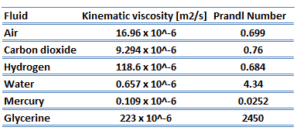 Small values of the Prandtl number, Pr << 1, means the thermal diffusivity dominates. Whereas with large values, Pr >> 1, the momentum diffusivity dominates the behavior. For example, the typical value for liquid mercury, which is about 0.025, indicates that the heat conduction is more significant compared to convection, so thermal diffusivity is dominant. When Pr is small, it means that the heat diffuses quickly compared to the velocity.
Small values of the Prandtl number, Pr << 1, means the thermal diffusivity dominates. Whereas with large values, Pr >> 1, the momentum diffusivity dominates the behavior. For example, the typical value for liquid mercury, which is about 0.025, indicates that the heat conduction is more significant compared to convection, so thermal diffusivity is dominant. When Pr is small, it means that the heat diffuses quickly compared to the velocity.
In comparison to Reynolds number, the Prandtl number is not dependent on geometry of an object involved in the problem, but is dependent solely on the fluid and the fluid state. As such, the Prandtl number is often found in property tables alongside other properties such as viscosity and thermal conductivity.
Prandtl Number of Water and Air
Air at room temperature has a Prandtl number of 0.71 and for water at 18°C it is around 7.56, which means that the thermal diffusivity is more dominant for air than for water. For a Prandtl number of unity, the momentum diffusivity equals the thermal diffusivity and the mechanism and rate of heat transfer are similar to those for momentum transfer. For many fluids, Pr lies in the range from 1 to 10. For gases, Pr is generally about 0.7.
Prandtl Number of Liquid Metals
For liquid metals the Prandtl number is very small, generally in the range from 0.01 to 0.001. This means that the thermal diffusivity, which is related to the rate of heat transfer by conduction, unambiguously dominates. This very high thermal diffusivity results from very high thermal conductivity of metals, which is about 100 times higher than that of water. The Prandtl number for sodium at a typical operating temperature in the Sodium-cooled fast reactors is about 0.004.
The Prandtl number enters many calculations of heat transfer in liquid metal reactors. Two of promising designs of Generation IV reactors use a liquid metal as the reactor coolant. The development of Generation IV reactors represents a challenge from an engineering point of view.
- Sodium-cooled fast reactor
- Lead-cooled fast reactor
One of the main challenges in numerical simulation is the reliable modeling of heat transfer in liquid-metal cooled reactors by Computational Fluid Dynamics (CFD). Heat transfer applications with low-Prandtl number fluids are often in the transition range between conduction and convection dominated regimes.
Laminar Prandtl Number – Turbulent Prandtl Number
When dealing with Prandtl numbers, we have to define a laminar part of Prandtl number and a turbulent part of Prandtl number. The equation Pr = ν/α , shows us actually only the laminar part which is only valid for laminar flows. The following equation shows us the effective Prandtl number:
Preff = ν/α + νt/αt
where νt is kinematic turbulent viscosity and αt is turbulent thermal diffusivity. The turbulent Prandtl number (Prt = νt/αt) is a non-dimensional term defined as the ratio between the momentum eddy diffusivity and the heat transfer eddy diffusivity. It simply describes mixing because of swirling/rotation of fluids. The simplest model for Prt is the Reynolds analogy, which yields a turbulent Prandtl number of 1.
In the special case where the Prandtl number and turbulent Prandtl number both equal unity (as in the Reynolds analogy), the velocity profile and temperature profiles are identical. This greatly simplifies the solution of the heat transfer problem. From experimental data, the turbulent Prandtl number is around 0.7 for different free shear layers, and for near-wall flows it is larger (Prt = 0.9) and occasionally beyond 1 since it has a tendency to grow larger when nearing the walls.
We hope, this article, Prandtl Number, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.